



The beginning of the month in the Babylonian calendar was determined by the direct observation by priests of the young crescent moon at sunset after the astronomical New Moon. This custom is remembered in Judaism and ʾIslām with the principle that the new calendar day begins at sunset. In ʾIslām, months whose commencement is of religious significance, like the month after the Fast of Ramadān, still depend on the actual observation of the crescent moon by a respected religious authority.
 The Months of the The Months of theBabylonian Calendar | |||||
|---|---|---|---|---|---|
| 1. Nisannu |  | 30 | 7. Tashritu |  | 30 |
| 2. Aiyaru |  | 29 | 8. Arakhsamna |  | 29 |
| 3. Simannu |  | 30 | 9. Kislimu |  | 30 |
| 4. Duʾuzu |  | 29 | 10. Ḍabitu |  | 29 |
| 5. Abu |  | 30 | 11. Sabaḍu |  | 30 |
| 6. Ululu I |  | 29 | 12. Addaru I |  | 29 |
| 6. Ululu II |  | 29 | 12. Addaru II |  | 30 |
If weather prevented the observation of the crescent, the Babylonians would begin the new month anyway after 30 days. In the Jewish and Islāmic calendars, each month is given a conventional length, alternating 30 days and 29 days. For convenience, the table at left applies that device for the Babylonian months, which will enable us to construct a working model of the Babylonian calendar without the priests of Marduk.
With the actual observation of the crescent by the Babylonians, eventually a pattern emerged, and this began to suggest a cycle. This was the 19 Year Cycle, discussed below. The cycle settled down into its classic form in the 19 year period beginning in 424 BC [R.A. Parker & W.H. Dubberstein, Babylonian Chronology, Providence, R.I., 1956]. A fairly complete record of intercalations is available from about 623. The distribution of intercalary months is evident from about 500, while the 424 cycle is noteworthy in that a second Ululu becomes standard in the 17th year. As it happens, the 17th year is the one in which Nisannu occurs the earliest.
 The Babylonian New Year was, astronomically, the first New Moon (actually the first visible crescent) after the Vernal Equinox. Modern dates on the Gregorian calendar for the Babylonian New Year may be chosen from the following table. The reckoning of the seasons by the Equinoxes and Solstices continues through the Greeks and Romans until present day astronomy.
The Babylonian New Year was, astronomically, the first New Moon (actually the first visible crescent) after the Vernal Equinox. Modern dates on the Gregorian calendar for the Babylonian New Year may be chosen from the following table. The reckoning of the seasons by the Equinoxes and Solstices continues through the Greeks and Romans until present day astronomy.
In the following table, the "uncorrected" dates use the 19 year lunar cycle, just as it was established in the 5th century BC, continued straight down to the present. The earliest New Year is marked with "<" and the latest with ">." Note that in the "uncorrected early" column the earliest date is only 3/31 and the latest is all the way to 4/28.
The 19 year cycle adjusts lunar months to the solar year; but if the Babylonian New Year was supposed to be the first New Moon after the Vernal Equinox, then the system has been running slow and the cycle is much in need of correction. There are no priests of Marduk any more to do that. The correction, however, can be accomplished simply by delaying every single intercalation a whole year. Hence the "corrected" columns, where earliest and latest dates are 3/20 & 4/17 (or 3/21 & 4/18).
 | Uncorrected | Corrected | |||
|---|---|---|---|---|---|
| AD/AN | AD/AN | early: | late: | early: | late: |
| 1990/2737 | 2009/2756 | 01- 4/26 | 01- 4/27 | 01* 3/27 | 01* 3/28 |
| 1991/2738 | 2010/2757 | 02- 4/15 | 02- 4/16 | 02- 4/15 | 02- 4/16 |
| 1992/2739 | 2011/2758 | 03* 4/4 | 03* 4/5 | 03- 4/4 | 03- 4/5 |
| 1993/2740 | 2012/2759 | 04- 4/23 | 04- 4/24 | 04* 3/24 | 04* 3/25 |
| 1994/2741 | 2013/2760 | 05- 4/12 | 05- 4/13 | 05- 4/12 | 05- 4/13 |
| 1995/2742 | 2014/2761 | 06* 4/1 | 06* 4/2 | 06- 4/1 | 06- 4/2 |
| 1996/2743 | 2015/2762 | 07- 4/20 | 07- 4/21 | 07* 3/21 | 07* 3/22 |
| 1997/2744 | 2016/2763 | 08* 4/9 | 08* 4/10 | 08- 4/9 | 08- 4/10 |
| 1998/2745 | 2017/2764 | 09- 4/28> | 09- 4/29> | 09* 3/29 | 09* 3/30 |
| 1999/2746 | 2018/2765 | 10- 4/17 | 10- 4/18 | 10- 4/17> | 10- 4/18> |
| 2000/2747 | 2019/2766 | 11* 4/6 | 11* 4/7 | 11- 4/6 | 11- 4/7 |
| 2001/2748 | 2020/2767 | 12- 4/25 | 12- 4/26 | 12* 3/26 | 12* 3/27 |
| 2002/2749 | 2021/2768 | 13- 4/14 | 13- 4/15 | 13- 4/14 | 13- 4/15 |
| 2003/2750 | 2022/2769 | 14* 4/3 | 14* 4/4 | 14- 4/3 | 14- 4/4 |
| 2004/2751 | 2023/2770 | 15- 4/22 | 15- 4/23 | 15* 3/23 | 15* 3/24 |
| 2005/2752 | 2024/2771 | 16- 4/11 | 16- 4/12 | 16- 4/11 | 16- 4/12 |
| 2006/2753 | 2025/2772 | 17§ 3/31< | 17§ 4/1< | 17- 3/31 | 17- 4/1 |
| 2007/2754 | 2026/2773 | 18- 4/18 | 18- 4/19 | 18§ 3/20< | 18§ 3/21< |
| 2008/2755 | 2027/2774 | 19* 4/7 | 19* 4/8 | 19- 4/7 | 19- 4/8 |
This was not even attempted by the Babylonians, so the table just provides a range (early vs. late), that we can compare with other lunar and luni-solar calendars. On the Moslem calendar the first day of the month is usually the second day after the astronomical New Moon (so that the crescent can be observed). The "late" columns fit that pretty well.
On the Jewish calendar, the first day of the month can be the New Moon itself, or it can be delayed as much as on the Moslem calendar. In 1992, for instance, both the Jewish and the Moslem months (Nîsân & Shawwâl) corresponding to the Babylonian New Year happen to begin on 4/4, only a day after the astronomical New Moon, so the "early" date would be preferable for the 1992 Babylonian New Year, lest 1 Nisannu be lonely on 4/5.
The "AN" years are the Era of Nabonassar, Anno Nabonassari, dating from the reign of the Babylonian King Nabûnâṣiru in 747 BC. Any AN year can be obtained simply by adding 747 to the year of the AD era. So the Babylonian New Year in 2016 AD, on April 9th (early), begins the year 2763 AN. Note that 747 BC is equivalent to -746 AD (1 BC=0 AD). The appropriate Seleucid year (Anno Seleucidarum), named after Seleucus I, one of Alexander the Great's generals, who obtained the eastern part of Alexander's Empire, can be calculated by adding 311 to the AD era -- e.g. 2016 AD = 2763 AN = 2327 Anno Seleucidarum -- but the Greek reckoning of 2327 begins the previous fall -- which is why a source like the Astronomical Almanac gives the Seleucid year for 2016 as 2328 (for September or October, or even July/August at Athens).
The Era of Nabonassar works excellently for the Babylonian calendar, since dividing any AN year by 19 gives the year of the 19 year cycle as the remainder; e.g. 2763/19 = 145 rem 8. Although the 19 year cycle was not regularized until the 4th century, the astronomical records handed down from the Babylonian Priests Kidunnu and Berossos through the Greco-Roman astronomer Claudius Ptolemy begin with Nabonassar. It was Ptolemy who thus formulated the Era of Nabonassar for his astronomical reckoning. The Era was never used by the Babylonians themselves.
A further complication was that the Era of Nabonassar was only used by Ptolemy in conjunction with the Egyptian calendar, which had a year that was exactly 365 days long (no leap years) and so ran fast: That "Era of Nabonassar" will already be up to 2765 AN in April 2016. Since Ptolemy lived in Egypt (in the days of Marcus Aurelius), the use of the Egyptian calendar was at hand, while he could derive his knowledge of the Babylonian calendar and astronomical data from the books by Kidunnu and Berossos, who wrote in Greek.
The Seleucid Era was used with the Babylonian calendar, but division by 19 inconveniently does not work with it. The Era of Nabonassar doesn't cover much of Mesopotamian history, but it does cover the history of the calendar that we know about; and Ptolemy's "Canon of Kings," a list of rulers from Nabonassar to the Roman Emperor Antoninus Pius, was absolutely fundamental for ancient chronology -- as recounted in E.J. Bickerman's Chronology of the Ancient World [Cornell, 1982].
The tables above are not constructed from astronomical data (except indirectly) but are schematically determined using a trick borrowed from the construction of the Gregorian Easter tables: the corresponding New Moon for the following year is determined simply by subtracting 11 from the given year's date; e.g. a 4/26 New Year one year means that the next year it will be 4/15. In an intercalary year (marked with "*"), 30 days are added; e.g. 4/4 -11 +30 = 4/23. This works out quite well, except that it comes out a day off after 19 years. The Gregorian Easter reckoning simply ignores that extra day.
With the Babylonian calendar, something else is possible: once every 19 years a second month of Ululu is added as the intercalary month instead of a second Addaru. Originally that was in the 17th year (marked "§"). If Ululu II is added as 29 days instead of 30, that makes the whole cycle come out even, which is what is done in the table. Year 17 also happens to be the one with the earliest New Year, so we could adopt the rule that the year with the earliest New Year, which will always be an intercalary year, is also the one with an extra Ululu instead of an extra Addaru. Hopefully, the priests of Marduk would have approved. In the "corrected" calendar, the year with Ululu II turns out to be year 18 anyway, which isn't very different from the traditional year.
Using Gregorian dates as above, we end up off by a day against the moon about every 235 years. Thus, as time goes on, a day must occasionally be added to the given dates. Right now we happen to be in a bit of a cusp: the "late" tables above will become increasingly accurate and will remain so for a couple of centuries, longer than we now need to worry about. Or we can simply construct a complete modern system for the Babylonian calendar, as follows.
Adding 7 months every 19 years approximates the solar year with 235 lunar months. That is mathematically (by  continued fractions) the most accurate convenient cycle for a luni-solar calendar and would give, using the mean value of the synodic month (29.530588 days), a year of 365.2467463 days long. This may be called the "Metonic" year, after the Greek astronomer who described the cycle, although the Babylonians discovered it first. The mean solar (tropical) year is 365.24219878 days long.
continued fractions) the most accurate convenient cycle for a luni-solar calendar and would give, using the mean value of the synodic month (29.530588 days), a year of 365.2467463 days long. This may be called the "Metonic" year, after the Greek astronomer who described the cycle, although the Babylonians discovered it first. The mean solar (tropical) year is 365.24219878 days long.
The calendar thus has two problems: (1) This is more accurate than the Julian Calendar (365.25) but less accurate than the Gregorian (365.2425) and must in the long run make provision for correction -- it is off a day every 219 years against the sun. (2) The calendar cannot be corrected for the sun by subtracting a day every 219 years or so, because this would then put it out of synchronization with the moon. A luni-solar calendar must regulate its lunar side with days and its solar side by its addition of months. The solar side thus must be corrected by modifying the 19 year cycle, most conveniently by delaying an intercalation every 342 years (18 cycles). By such delays, the calendar would lose an entire month after 6498 years, which reduces the Metonic year to 365.2422018 days, accurate to a day in 336,700 years.
For the moon, days may be added just as days are added to the Julian, Gregorian, and Moslem calendars. The Julian pattern, a day every four years, is conveniently accurate, more accurate than in the Julian calendar itself: 365.25 days is off a day in 307 years against the Metonic year but off a day in only 128 years against the solar year [note that the Gregorian year, 365.2525, is less accurate against the Metonic year, off a day in 235 years]. A Gregorian-like correction on the Julian year may thus be imposed against the Metonic year: skipping a day every 300 years; 365 + 1/4 - 1/300 = 365.246. That approximates the Metonic year to within a day every 12,555 years. Quite accurate enough for the moon.
With the 6498 year cycle of intercalations, 365 +1/4 - 1/300 - 29/6498, this produces a solar year of 365.2422038 days. That is not quite as accurate as the pure intercalation cycle: it is now off a day in 201,005 years. That is practically perfect, however; the orbits of the earth and the moon are liable to vary enough in that period of time, and the rotation of the earth to slow down enough, to render greater "accuracy" meaningless.
The 6498 year cycle interacts curiously with the Jewish and Christian Eras of the World that have been calculated by traditional religous scholars.

Babylonian Numbers and Measure
 The Jewish and Moslem Calendars with the Era of Nabonassar
The Jewish and Moslem Calendars with the Era of Nabonassar
Philosophy of Science, Calendars
Philosophy of History, Calendars

 The Jewish and Moslem Calendars
The Jewish and Moslem Calendars
and the Era of Nabonassar
 Jewish JewishMonths | Christian Months in Arabic | ||
|---|---|---|---|
1.  Tišrî | 30 | 10.  Tišrînu l-ʾawwâl | October |
2.  Ḫešwân | 29/30 | 11.  Tišrînu θ-θânî | November |
3.  Kislêw | 30/29 | 12.  Kânûnu l-ʾawwâl | December |
4.  Ṭêbêt | 29 | 1.  Kânûnu θ-θânî | January |
5.  Šebâṭ | 30 | 2.  Šubâṭ | February |
6.  ʾAdâr | 29 | 3.  ʾÂðâr | March |
6.   ʾAdâr Šênî | 30 | No intercalations | |
7.  Nîsân | 30 | 4.  Nîsân | April |
8.  ʾIyyâr | 29 | 5.  ʾAyyâr | May |
9.  Sîwân | 30 | 6.  Ḥazîrân | June |
10.  Tammûz | 29 | 7.  Tammûz | July |
11.  ʾÂb | 30 | 8.  ʾÂb | August |
12.  ʾElûl | 29 | 9.  ʾAylûl | September |
The 19 year cycle is the only true cycle in the Jewish calendar, since the method of adding days depends on the mean value of the synodic month and does not produce a repetition of dates within any significant length of time. The dates of Rôʾš Haššānâh, however, roughly repeat after 247 years (13 cycles).
The names of the Babylonian months are retained not only in the Jewish calendar but in the Gregorian calendar used by Christians in the Levant and Iraq. The Arabic versions of these names are given in the table at right. There have been some alterations, with three of the ancient names dropped, a new one --  , Kânûn -- introduced, and two of the names used twice. I have not seen an explanation for these alterations. In Egypt we see Arabic versions of the familiar names from Latin.
, Kânûn -- introduced, and two of the names used twice. I have not seen an explanation for these alterations. In Egypt we see Arabic versions of the familiar names from Latin.
The Moslem calendar consists of years of 12 lunar months. A reform effected by the Prophet Muḥammad dispenses with attempts at intercalation. The Moslem year is therefore short, only 354 or 355 days, and the calendar runs fast. The Era for the calendar begins on the evening of the Prophet's Flight from Mecca to Medina. That occurred at the time of the first visible crescent of the New Moon, on the first day of the month of Muḥarram, or 16 July 622 AD (Julian reckoning). The "Flight" in Arabic is the  , Hijrah, so the Era of the Moslem calendar is called that of the Hijrah or, in English, the Hegira -- "AH," the Anno Hegirae.
, Hijrah, so the Era of the Moslem calendar is called that of the Hijrah or, in English, the Hegira -- "AH," the Anno Hegirae.
The problem of the Moslem clalendar is then simply to add days to keep it accurate with the moon.
 The Months of the The Months of theMoslem Calendar | |||
|---|---|---|---|
1.  ʾal-Muḥarram | 30 | 7.  Rajab | 30 |
2.  Ṣafar | 29 | 8.  Šaʿbân | 29 |
3.  Rabîʿu l-ʾawwal | 30 | 9.  Ramaḍân | 30 |
4.  Rabîʿu θ-θânî | 29 | 10.  Šawwâl | 29 |
5.  Jumâdâ l-ʾûlâ | 30 | 11.  Ðû l-Qaʿdah | 30 |
6.  Jumâdâ l-ʾâḫirah | 29 | 12.  Ðû l-Ḥijjah | 29/30 |
While the Islamic "reform" of the calendar can be criticized for detaching it from the seasons, this is actually an advantage when the primarily ritual use of the calendar is remembered. The month of Ramaḍân, when believers fast the daylight hours, is much less of a hardship in the winter than in the summer. Were Ramaḍân always in the summer, this would, of course, be in the Northern Hemisphere, which means that Moslems could moderate the demands of their religion by moving to South Africa, Australia, or Argentina, where summer in the Northern Hemisphere corresponds to winter in the Southern. Instead, since Ramaḍân moves through the seasons, the fast will occur in both winter and summer, separated by about 16 years, in every location. The remaining hardship will only be for those stationed at the South Pole during summer, when there is constant daylight for six months. However, there are provisions for this in Islamic Law, where such a hardship, which would result in continuous (and fatal) months of fasting, warrants a postponement of the fast to more appropriate circumstances.

 Since the Jewish calendar adds a month every two or three years, the correspondence between Jewish and Moslem months shifts at those times. Muḥarram of year 1 of the Hegira Era corresponded to Ab in the Jewish calendar. Muḥarram moves entirely around through the seasons and returns to being Ab in 32 or 33 years. If we ask how long it would take for the 19 year Jewish cycle and the 30 year Moslem cycle to commensurate, this turns out to be 1368 solar (Jewish) or 1410 Moslem years. The table below left shows how these numbers break down into prime (or small multiples of prime) factors.
Since the Jewish calendar adds a month every two or three years, the correspondence between Jewish and Moslem months shifts at those times. Muḥarram of year 1 of the Hegira Era corresponded to Ab in the Jewish calendar. Muḥarram moves entirely around through the seasons and returns to being Ab in 32 or 33 years. If we ask how long it would take for the 19 year Jewish cycle and the 30 year Moslem cycle to commensurate, this turns out to be 1368 solar (Jewish) or 1410 Moslem years. The table below left shows how these numbers break down into prime (or small multiples of prime) factors.
| 1368 y |  = 16920 m | ||||
|---|---|---|---|---|---|
| 235 m | x 72 | ||||
| 47 | x 5 | x 6 | x 4 | x 3 | |
| x 30 y | x 12 m | ||||
| 1410 y | |||||
1368 is a number that turns out to have a curious property. 1368 years before 622 AD puts us in 747 BC, the first year of the Era of Nabonassar, which was used by Claudius Ptolemy for his system of chronology. An interesting coincidence. The year 1 AH is thus the year 1369 AN. The full Jewish/Moslem cycle brings us from 622 AD down into our own time: 622 plus 1368 is 1990. The year 1990 thus corresponds to 1411 AH and to 2737 (1368 x 2 + 1) AN.
It may also be worth noting that the oldest Greek text of Ptolemy's Almagest was a gift from the Emperor Manuel I (1143-1180) to King William I (1154-1166) of Sicily, whence it eventually passed to Venice, where it remains.
The numerical coincidences with 1368 may be of no practical importance, but it is a curiosity of history that the Era of a Babylonian King, as used by a Greco-Roman astronomer with the Egyptian calendar, fits in with the Era of the Moslem calendar on the basis of a cycle generated by the interaction of the Islâmic calendar 30 year cycle and the Babylonian 19 year cycle as used by the Jewish calendar.
| Jewish Months |  ʾal-Muḥarram |  Years AD Years AD | |
|---|---|---|---|
1.  Tišrî | 30 | 1438, 1439, 1440 AH | 2016, 2017, 2018 AD |
2.  Ḫešwân | 29/30 | 1436, 1437 AH | 2014, 2015 AD |
3.  Kislêw | 30/29 | 1433, 1434, 1435 AH | 2011, 2012, 2013 AD |
4.  Ṭêbêt | 29 | 1430, 1431, 1432 AH | 2008, 2009, 2010 AD |
5.  Šebâṭ | 30 | 1427, 1428, 1429 AH | 2006, 2007, 2008 AD |
6.  ʾAdâr | 29 | 1425, 1426 AH | 2004, 2005 AD |
6.   ʾAdâr Šênî; 30 | 1424 AH | 2003 AD | |
7.  Nîsân | 30 | 1421, 1422, 1423 AH | 2000, 2001, 2002 AD |
8.  ʾIyyâr | 29 | 1418, 1419, 1420 AH | 1997, 1998, 1999 AD |
9.  Sîwân | 30 | 1416, 1417 AH | 1995, 1996 AD |
10.  Tammûz | 29 | 1414, 1415, 1446 AH | 1993, 1994, 2024 AD |
11.  ʾÂb | 30 | 1444, 1445 AH | 2022, 2023 AD |
12.  ʾElûl | 29 | 1441, 1442, 1443 AH | 2019, 2020, 2021 AD |
When I discovered the 1368 year match between Jewish and Moslem calendars, then that it was exactly 1368 years from the reign of Nabonassar to the date of the Hijrah, and then that 1990 would begin a new cycle -- all in, as I recall, 1978 -- it all seemed magical beyond coincidence. As it happens, 1368 is also, on Christian reckoning, the first year of the Ming Dynasty in China -- a date extraneous to Chinese reckoning but nevertheless nicely memorable in the "Common Era," as the international use of Annō Domini dating has come to be termed. Nabonassar would perhaps have much less baggage as a proper "Common Era."
However magical, and if I were really open to numerology, the number 1368 otherwise, I am sorry to say, seems to have little import, use, or meaning. It remains confined to this remarkable calendrical coincidence. See how the Era of Nabonassar can be used for a Modern Luni-Solar Calendar.
Meanwhile, the table at right keeps track of the months in the Jewish calendar to which the Islâmic month of Muḥarram corresponds, from 1993 to 2024 -- where in both years Muḥarram corresponds to Tammûz. Some features are noteworthy. One is that, since months are added every two or three years to the Jewish calendar, we see sometimes two years, sometimes three, where Muḥarram matches a particular month. Another feature is that in 2003 Muḥarram corresponded to the intercalary month itself. This can only happen in one year, since in the next year that month is gone. Third, there is one year, 2008, where Muḥarram actually occurs twice, but matching different Jewish months. Obviously, an intercalation must have occurred in between them. Since the Common Islâmic year is only 354 days long, a 365 day solar year will occasionally include two Islâmic New Years. Finally, in 2016, 2017, and 2018, the Jewish and Islâmic New Years coincided. I don't know if any combined celebrations were held, but it would have been nice.

Islâmic Dates with Julian Day Numbers
Philosophy of Religion, Calendars
Philosophy of History, Calendars
Information about the origin of the modern Jewish calendar is not always historically accurate. It is often said that the calendar was formulated by Patriarch Hillel II in 358/359 AD/CE. However, it appears likely that the calendar reform at this point was simply to introduce the Babylonian 19 year cycle, which meant that lunar intercalations did not need to be announced year by year. We can estimate the date for the present full mechanism of the calendar from the amount of error that has accumulated. If we run things back to when it would have been accurate for a meridian through Jerusalem or Babylon, the centers of Jewish life and calendar studies, we just get back to around the 9th or 10th centuries. As it happens, we know that there were controversies about the calendar in that era. Saʿadîah Gaʾôn (882-942), who wrote works on the calendar, participated in a dispute about whether the Palestinian or Babylonian communities would rule on calendar issues. He represented the Babylonian community (which by then centered more in Baghdad, where recourse was sometimes needed to rulings by the Caliph, than in Babylon, which had already been found abandoned by the Emperor Trajan), which won the dispute. It seems beyond coincidence that was the period for which the new Moon benchmark would have been accurate, and it implies a Babylonian meridian.
The following technique for analyzing the Jewish calendar is based on that of Charles Kluepfel, known from personal correspondence (back in the 1970's, thanks to our mutual friend O.L. Harvey), with definitions paraphrased from Arthur Spier, The Comprehensive Hebrew Calendar (Feldheim Publishers, 1986).
The date of Rōʾš Haššānâh is determined by the occurrence of the actual mean New Moon, the If the reckoning of days is always kept to whole weeks following an original Shabbâth, the remaining excess of parts places the Môlâd Tišrî in a clear relation to the week. In the following tables for the determination of 
 The Jewish Calendar
The Jewish Calendar
 The benchmark for the New Moon is now accurate for a meridian in Afghanistan.
The benchmark for the New Moon is now accurate for a meridian in Afghanistan.
 , Môlâd ("birth"), associated with the first month of the year, Tišrî. Calculated to an accuracy of 3/10 of a minute, the length of the synodic month is expressed in special units (at 18/minute or 1080/hour) called
, Môlâd ("birth"), associated with the first month of the year, Tišrî. Calculated to an accuracy of 3/10 of a minute, the length of the synodic month is expressed in special units (at 18/minute or 1080/hour) called  , Ḫalâqîm (singular
, Ḫalâqîm (singular  , ḫêleq), "parts" (p). The synodic month (m) is thus 765433p long. The day is considered to begin at mean sunset or 6 PM. Noon is therefore reckoned to occur at 18h, not 12h. The Môlâd Tišrî is calculated by an absolute counting of months from a Benchmark of 5h 204p on Monday 7 October 3761 BC/BCE (the Môlâd Tišrî of year 1 Annō Mundi).
, ḫêleq), "parts" (p). The synodic month (m) is thus 765433p long. The day is considered to begin at mean sunset or 6 PM. Noon is therefore reckoned to occur at 18h, not 12h. The Môlâd Tišrî is calculated by an absolute counting of months from a Benchmark of 5h 204p on Monday 7 October 3761 BC/BCE (the Môlâd Tišrî of year 1 Annō Mundi).

 , Rōʾš Haššānâh (the "Head of the Year"), only the excess of parts need be stated. However, for the determination of an absolute date in relation to other calendars, a count of whole weeks and excess parts may be made for convenience from a 0 AM year benchmark (3762 BC or -3761 AD) of Julian Date 347,610d, with an excess of 60,095p. The four deḫiyyôth or postponements modify the way in which the Môlâd Tišrî determines Rôʾš Haššānâh. Note that since a zero year benchmark is used, Rôʾš Haššānâh for the year 1 AM must be calculated with additions and subtractions just as for other years.
, Rōʾš Haššānâh (the "Head of the Year"), only the excess of parts need be stated. However, for the determination of an absolute date in relation to other calendars, a count of whole weeks and excess parts may be made for convenience from a 0 AM year benchmark (3762 BC or -3761 AD) of Julian Date 347,610d, with an excess of 60,095p. The four deḫiyyôth or postponements modify the way in which the Môlâd Tišrî determines Rôʾš Haššānâh. Note that since a zero year benchmark is used, Rôʾš Haššānâh for the year 1 AM must be calculated with additions and subtractions just as for other years.
| 247 Year Cycles | ||||
|---|---|---|---|---|
| y AD | y AM | JD | d | p |
| -3761 | 0 | 347,610 | 60,095 | |
| -- | ||||
| 933 | 4693 | 2061,714 | 42,900 | |
| 1180 | 4940 | 2151,930 | 41,995 | |
| 1427 | 5187 | 2242,146 | 41,090 | |
| 1674 | 5434 | 2332,362 | 40,185 | |
| 1921 | 5681 | 2422,578 | 39,280 | |
| 2168 | 5928 | 2512,794 | 38,375 | |
| 247 Year Cycle | ||
|---|---|---|
| y | d | p |
| 0 | 0 | 0 |
| 19 | 6,937 | 69,715 |
| 38 | 13,874 | 139,430 |
| 57 | 20,818 | 27,705 |
| 76 | 27,755 | 97,420 |
| 95 | 34,692 | 167,135 |
| 114 | 41,636 | 55,410 |
| 133 | 48,573 | 125,125 |
| 152 | 55,517 | 13,400 |
| 171 | 62,454 | 83,115 |
| 190 | 69,391 | 152,830 |
| 209 | 76,335 | 41,105 |
| 228 | 83,272 | 110,820 |
| 247 | 90,209 | 180,535 |
Next we move on to the table, at right, that breaks down the 247 year cycle.
| The 19 Year Cycle | |||
|---|---|---|---|
| y | d | p | type |
| 0* | 0 | 0 | L |
| 1 | 378 | 152,869 | CF |
| 2 | 735 | 84,625 | C |
| 3* | 1092 | 16,381 | L |
| 4 | 1470 | 169,250 | CF |
| 5 | 1827 | 101,006 | C |
| 6* | 2184 | 32,762 | L |
| 7 | 2569 | 4,191 | CB |
| 8* | 2919 | 117,387 | L |
| 9 | 3304 | 88,816 | CF |
| 10 | 3661 | 20,572 | C |
| 11* | 4011 | 133,768 | L |
| 12 | 4396 | 105,197 | CF |
| 13 | 4753 | 36,953 | C |
| 14* | 5103 | 150,149 | L |
| 15 | 5488 | 121,578 | CF |
| 16 | 5845 | 53,334 | C |
| 17* | 6195 | 166,530 | L |
| 18 | 6580 | 137,959 | CB |
| 19* | 6937 | 69,715 | L |
If our sum of parts ends up being larger than the number of parts in a week, we must substract 181,440p from the total and add 7d to the sum of days. If the sum of parts is still larger than a week, we must repeat the procedure. However, in this case, for 5771 AM, our sum of parts is below 181,440p and this procedure is unncessary.
Now we must locate ourselves within the 19 year cycle, which is displayed on the table at left. The remainder of years above was 14y, so we are in the 14th year of the 19 year cycle. We see from the table that this is a Leap Year (L). This of significance below. Meanwhile, we must add the days and parts for year 14 to our running sums: 2450,333d + 5103 = 2455,436d and 136,700 + 150,149 = 286,849p.
| w | p | d |
|---|---|---|
| 2 | 362,880 | 14 |
| 1 | 181,440 | 7 |
We now have reduced the year count to zero and have the obtained values 2455,443d 105,409p for the year 5771 AM. Since this is for day zero of 5771 AM, we would actually need to add 1d to get the Julian Day Number for Rôʾš Haššānâh. But it is not going to be that simple. For four reasons Rôʾš Haššānâh can be delayed. These are the  , deḫiyyôth, "postponements" (singular
, deḫiyyôth, "postponements" (singular  , deḫiyyâh), and our calculation of the Day Number must be postponed until the effects of the deḥiyyôth are examined. Note in the following tables, however, that the number of the day of the week (e.g. 1 for Sunday) will be a number that we add to the total for days, as well as the day of the month (e.g. 1 for 1 Tišrî). The number of parts in our sum places us in the week of Rôʾš Haššānâh. The number of parts in our sum is not subsequently altered.
, deḫiyyâh), and our calculation of the Day Number must be postponed until the effects of the deḥiyyôth are examined. Note in the following tables, however, that the number of the day of the week (e.g. 1 for Sunday) will be a number that we add to the total for days, as well as the day of the month (e.g. 1 for 1 Tišrî). The number of parts in our sum places us in the week of Rôʾš Haššānâh. The number of parts in our sum is not subsequently altered.
| The First Deḫiyyâh | |||||
|---|---|---|---|---|---|
| Before | d | p | After | d | p |
| Sunday | 1 | 0 | 0 | ||
| Monday | 2 | 25,920 | Monday | 2 | |
| Tuesday | 3 | 51,840 | Tuesday | 3 | 51,840 |
| Wednesday | 4 | 77,760 | 77,760 | ||
| Thursday | 5 | 103,680 | Thursday | 5 | |
| Friday | 6 | 129,600 | 129,600 | ||
| Shabbât | 7 | 155,520 | Shabbât | 7 | |
| Sunday | 8 | 181,440 | 181,440 | ||
| Monday | 9 | Monday | 9 | ||
When the Môlâd Tišrî occurs on a Sunday, Wednesday, or Friday -- using the thresholds in relation to our sum of parts -- Rôʾš Haššānâh is postponed to the following day. This is done to prevent Yôm Kippûr from occurring on the day before or the day after the Shabbâth or Hôshanâ Rabbâ from occurring on the Shabbât.
To construct the table, we add 25,920p (1080p x 24h) for each day; strike out disallowed days and irrelevant thresholds. Sunday and Monday of the following week are included in this table for reasons that will be apparent in the second deḥiyyâh.
| The First Deḫiyyâh, Closed Up | d | p |
|---|---|---|
| Monday | 2 | 0 |
| Tuesday | 3 | 51,840 |
| Thursday | 5 | 77,760 |
| Shabbât | 7 | 129,600 |
| Monday | 9 | 181,440 |
When the Môlâd Tišrî occurs at noon (18h) or later, Rôʾš Haššānâh is postponed to the next day -- or if this day is a Sunday, Wednesday, or Friday, to Monday, Thursday, or the Shabbât, respectively, because of the first deḥiyyâh. This is done to prevent Rôʾš Haššānâh from occurring before the New Moon, since the reckoning of the Môlâd is based
| The Second Deḫiyyâh | |||||
|---|---|---|---|---|---|
| Before | d | p | After | d | p |
| Monday | 2 | 0 | Monday | 2 | 0 |
| Tuesday | 3 | 51,840 | Tuesday | 3 | 45,360 |
| Thursday | 5 | 77,760 | Thursday | 5 | 71,280 |
| Shabbât | 7 | 129,600 | Shabbât | 7 | 123,120 |
| Monday | 9 | 181,440 | Monday | 9 | 174,960 |
To construct the table, subtract 6480p (=6h) from each threshold. We now notice that the threshold for the following Monday is within the range of our current week (181,440p).
| Common Years (C) (see below for common years following leap years) | |||
|---|---|---|---|
| Before Third Deḫiyyâh | After Third Deḫiyyâh (C) | ||
| 2/353/5 | 0 + 113,196 = 113,196 | 2/353/5 | 0 + 113,196 = 113,196 |
| 2/355/7 | 9,924 = 123,120 - 113,196 | 2/355/7 | 9,924 = 123,120 - 113,196 |
| 3/354/7 | 45,360 + 113,196 = 158,556 | 3/354/7 | 45,360 + 113,196 = 158,556 |
| 3/356/2 | 61,764 = 174,960 - 113,196 | 61,764 = 174,960 - 113,196 | |
| 5/354/2 | 71,280 + 113,196 = 3,036 | 5/354/2 | |
| 5/355/3 | 113,604 = 45,360 - 113,196 | 5/355/3 | 113,604 = 45,360 - 113,196 |
| 7/353/3 | 123,120 + 113,196 = 54,876 | 7/353/3 | 123,120 + 113,196 = 54,876 |
| 7/355/5 | 139,524 = 71,280 - 113,196 | 7/355/5 | 139,524 = 71,280 - 113,196 |
| 9/353/5 | 174,960 + 113,196 = 106,446 | 9/353/5 | 174,960 + 113,196 = 106,446 |
When the Môlâd Tišrî of a common year falls on Tuesday, 204 parts after 3 A.M. (3d 9h 204p or 61,764p) or later, Rôʾš Haššānâh is postponed to Wednesday, and, because of the first deḥiyyâh, further postponed to Thursday (5/354/2). This is done to eliminate a common year that is 356d long, making for only seven kinds of common year. Drop the year 3/356/2 and the irrelevant old threshold for Thursday. Now there is a single threshold for the change from Tuesday to Thursday for the current year and from Saturday to Monday for the following year.
| Leap Years (L) | |||
|---|---|---|---|
| Before Fourth Deḫiyyâh | After Fourth Deḫiyyâh (L) | ||
| 2/383/7 | 0 + 152,869 = 152,869 | 2/383/7 | 0 + 152,869 = 152,869 |
| 2/385/2 | 22,091 = 174,960 - 152,869 | 2/385/2 | 22,091 = 174,960 - 152,869 |
| 3/384/2 | 45,360 + 152,869 = 16,789 | 3/384/2 | 45,360 + 152,869 = 16,789 |
| 5/382/2 | 71,280 + 152,869 = 42,709 | 71,280 + 152,869 = 42,709 | |
| 5/383/3 | 73,931 = 45,360 - 152,869 | 5/383/3 | |
| 5/385/5 | 90,335 = 61,764 - 152,869 | 5/385/5 | 90,335 = 61,764 - 152,869 |
| 7/383/5 | 123,120 + 152,869 = 94,549 | 7/383/5 | 123,120 + 152,869 = 94,549 |
| 7/385/7 | 151,691 = 123,120 - 152,869 | 7/385/7 | 151,691 = 123,120 - 152,869 |
| 9/383/7 | 174,960 + 152,869 = 146,389 | 9/383/7 | 174,960 + 152,869 = 146,389 |
| Common Years between leap years (CB) | |||
|---|---|---|---|
| Before Fourth Deḫiyyâh (C) | After Fourth Deḫiyyâh (CB) | ||
| 2/353/5 | 0 + 113,196 = 113,196 | 2/353/5 | 0 + 113,196 = 113,196 |
| 2/355/7 | 9,924 = 123,120 - 113,196 | 2/355/7 | 9,924 = 123,120 - 113,196 |
| 3/354/7 | 45,360 + 113,196 = 158,556 | 3/354/7 | 42,709 + 113,196 = 155,905 |
| 5/354/2 | 61,764 + 113,196 = 174,960 | 5/354/2 | 61,764 + 113,196 = 174,960 |
| 5/355/3 | 113,604 = 45,360 - 113,196 | 5/355/3 | 113,604 = 45,360 - 113,196 |
| 7/353/3 | 123,120 + 113,196 = 54,876 | 7/353/3 | 123,120 + 113,196 = 54,876 |
| 7/355/5 | 139,524 = 71,280 - 113,196 | 7/355/5 | 139,524 = 71,280 - 113,196 |
| 9/353/5 | 174,960 + 113,196 = 106,446 | 9/353/5 | 174,960 + 113,196 = 106,446 |
| Common Years following but not between leap years (CF) | |||
|---|---|---|---|
| Before Fourth Deḫiyyâh (C) | After Fourth Deḫiyyâh (CF) | ||
| 2/353/5 | 0 + 113,196 = 113,196 | 2/353/5 | 0 + 113,196 = 113,196 |
| 2/355/7 | 9,924 = 123,120 - 113,196 | 2/355/7 | 9,924 = 123,120 - 113,196 |
| 3/354/7 | 45,360 + 113,196 = 158,556 | 3/354/7 | 42,709 + 113,196 = 155,905 |
| 5/354/2 | 61,764 + 113,196 = 174,960 | 5/354/2 | 61,764 + 113,196 = 174,960 |
| 5/355/3 | 113,604 = 45,360 - 113,196 | 5/355/3 | 113,604 = 45,360 - 113,196 |
| 7/353/3 | 123,120 + 113,196 = 54,876 | 7/353/3 | 123,120 + 113,196 = 54,876 |
| 7/355/5 | 139,524 = 71,280 - 113,196 | 7/355/5 | 130,008 = 61,764 - 113,196 |
| 9/353/5 | 174,960 + 113,196 = 106,446 | 9/353/5 | 174,960 + 113,196 = 106,446 |
This may all seem fearfully confusing. Traditionally it has been the principle matter of concern for the calculation of Rôʾš Haššānâh. Here, however, the results may be simplified for our purposes.

| Results of all the Deḫiyyôth | |||||||||
|---|---|---|---|---|---|---|---|---|---|
| L p | d | d/y | f | CF p | CB p | C p | d | d/y | f |
| 0 | 2 | 383 | 7 | 0 | 0 | 0 | 2 | 353 | 5 |
| 22,091 | 2 | 385 | 2 | 9,924 | 9,924 | 9,924 | 2 | 355 | 7 |
| 45,360 | 3 | 384 | 2 | 42,709 | 42,709 | 45,360 | 3 | 354 | 7 |
| 71,280 | 5 | 383 | 3 | 61,764 | 61,764 | 61,764 | 5 | 354 | 2 |
| 90,335 | 5 | 385 | 5 | 113,604 | 113,604 | 113,604 | 5 | 355 | 3 |
| 123,120 | 7 | 383 | 5 | 123,120 | 123,120 | 123,120 | 7 | 353 | 3 |
| 151,691 | 7 | 385 | 7 | 130,008 | 139,524 | 139,524 | 7 | 355 | 5 |
| 174,960 | 9 | 383 | 7 | 174,960 | 174,960 | 174,960 | 9 | 353 | 5 |
The process of converting from Julian Day Numbers to Julian or Gregorian dates is examined elsewhere. Or the Gregorian date may be read from an almanac, for instance The Astronomical Almanac for the Year 2010 [U.S. Government Printing Office, Washington, and Her Majesty's Stationery Office, London, 2008]. There on page B18, we find the "Julian Date" for 9 September given as "5448.5." This is the number in "myriads," i.e. four integers before the decimal, leaving out the "245" of the full count. Also, the "Julian Date" is for the day count at Midnight of 9 September. The proper Julian Day Number, 2454,449d, is for the following Noon, which is the beginning of the Julian Day, as before 1925 Noon was the beginning of the Nautical and Astronomical days.
For Common years, the process of using the table works the same way, except that we must select the appropriate column for the three different kinds of common years, i.e. following or between leap years, or neither. For instance, we can examine the calculation for last year, 5770 AM. The day sum looks like this: 2422,578 + 27,755 + 4753 = 2455,086d and 39,280 + 97,420 + 36,953 = 173,653p. 5770 AM is year 13 in the 19 year cycle, a Common (C) year. The part sum exceeds the threshold (139,524) for a Common year 7/355 (7/355/5, which gives us the day of the week for Rôʾš Haššānâh of 5771). The day sum this thus 2455,086 + 7 + 1 = 2455,094d, which is Saturday, 19 September 2009.
 Jewish JewishMonths | Common Years | Leap Years | |||||
|---|---|---|---|---|---|---|---|
| 353 | 354 | 355 | 383 | 384 | 385 | ||
1.  , Tišrî , Tišrî | 30 | 0 | 0 | 0 | 0 | 0 | 0 |
2.  ( ( ), ),(Mar)Ḫešwân | 29/30 | 30 | 30 | 30 | 30 | 30 | 30 |
3.  , Kislêw , Kislêw | 30/29 | 59 | 59 | 60 | 59 | 59 | 60 |
4.  , Ṭêbêt , Ṭêbêt | 29 | 88 | 89 | 90 | 88 | 89 | 90 |
5.  , Shebâṭ , Shebâṭ | 30 | 117 | 118 | 119 | 117 | 118 | 119 |
6.  , ʾAdâr , ʾAdâr | 29 | 147 | 148 | 149 | 147 | 148 | 149 |
6.   , ,ʾAdâr Šênî | 30 | 177 | 178 | 179 | |||
7.  , Nîsân , Nîsân | 30 | 176 | 177 | 178 | 206 | 207 | 208 |
8.  , ʾIyyâr , ʾIyyâr | 29 | 206 | 207 | 208 | 236 | 237 | 238 |
9.  , Sîwân , Sîwân | 30 | 235 | 236 | 237 | 265 | 266 | 267 |
10.  , Tammûz , Tammûz | 29 | 265 | 266 | 267 | 295 | 296 | 297 |
11.  , ʾÂb , ʾÂb | 30 | 294 | 295 | 296 | 324 | 325 | 326 |
12.  , ʾElûl , ʾElûl | 29 | 324 | 325 | 326 | 354 | 355 | 356 |
For 5771 AM, what we do then is the addition 2455,443 + 5 + [month of 385d year] + [day of month] = [Julian Day Number].
Lost in all our calcuations may be a characteristic of the Annō Mundi date. If we divide the Annō Mundi year by 19, the remainder gives us the position of the year in the 19th year cycle. Thus, 5771/19 = 303 remainder 14. This works like the years of the Era of Nabonassar for the Babylonian calendar. 5771 AM corresponds to 2758 AN -- 2758/19 = 145 remainder 3.
There may be grounds for some confusion here, since 2758 AN does not begin until April 2011. However, the years of the Era that was actually used with the Babylonian calendar, the Seleucid Era, where 2758 AN is 2322 Annō Seleucidae, was reckoned by the Greeks from the previous Autumn. So 2322 ASel clearly corresponds to 5771 AM, and that is the basis of matching it with 2758 AN, even though the latter begins by Babylonian reckoning six months later.
The same year in the Jewish and Babylonian calendars is at different places in the 19 year cycle because the two cycles are out of phase. This happened because of the error that had built up over the centuries in the Babylonian calendar. The Jewish calendar of the time of Saddiah Goan has corrected the intercalation of months. The pattern of Babylonian intercalations is preserved by starting the cycle at a different point. Of course, since then, error has built up in the Jewish calendar. The 8th and 19th year leap years, at least, should be delayed one year. This would require changing the traditional pattern of intercalations, which has never been done. The existence of a center of Jewish life and religious authority, in Israel, however, does mean that the calendar could be authoritatively reformed, even if the priests of the Sanhedrîn, who originally governed the calendar, do not exist. The chief Rabbis of the Sephardic and Ashkenazic communities could easily assume that responsibility.

The calculation for AM 5772 begins with the same numbers for the benchmark (5681y 2422,578d 39,280p) and the 247 year cycle (76y 27,755d 97,420p). Now however, we are in the 15th year of the 19 year cycle (15y 5488d 121,578p). This will be a Common Year following a Leap Year (CF). Adding the numbers gives a total of 2455 821d 258 278p. The number of parts exceeds a week, so we subtract 181 440p and add 7d: 2455 828d 76 838p. When we examine the table for the excess of parts, we are over the threshold at 61,764p, which gives us a year 5/354/2, a year that begins on a Thursday, is 354 days long, and will be followed by a year (5773) that begins on a Monday. Thus, for the final Julian Day Number, we add 5d for Thursday and 1d for 1 Tišrî to our total of days: JD 2455 834d. This turns out to be 29 September 2011.

Like 5772, the calculation for AM 5773 begins with the same numbers for the benchmark (5681y 2422,578d 39,280p) and the 247 year cycle (76y 27,755d 97,420p). Now we are in the 16th year of the 19 year cycle (16y 5845d 53,334p). This will be a Common Year (C). Adding the numbers gives a total of 2456 178d 190 034p. The number of parts exceeds a week, so we subtract 181 440p and add 7d: 2456 185d 8 594p. When we examine the table for the excess of parts, we are not over the threshold at 9,924p, which gives us a year 2/353/5, a year that begins on a Monday, is 353 days long, and will be followed by a year (5774) that begins on a Thursday. Thus, for the final Julian Day Number, we add 2d for Monday and 1d for 1 Tišrî to our total of days: JD 2456 188d. This turns out to be 17 September 2012.

| Results of all the Deḫiyyôth, 5777 | |||||||||
|---|---|---|---|---|---|---|---|---|---|
| L p | d | d/y | f | CF p | CB p | C p | d | d/y | f |
| 0 | 2 | 383 | 7 | 0 | 0 | 0 | 2 | 353 | 5 |
| 22,091 | 2 | 385 | 2 | 9,924 | 9,924 | 9,924 | 2 | 355 | 7 |
| 45,360 | 3 | 384 | 2 | 42,709 | 42,709 | 45,360 | 3 | 354 | 7 |
| 71,280 | 5 | 383 | 3 | 61,764 | 61,764 | 61,764 | 5 | 354 | 2 |
| 90,335 | 5 | 385 | 5 | 113,604 | 113,604 | 113,604 | 5 | 355 | 3 |
| 123,120 | 7 | 383 | 5 | 123,120 | 123,120 | 123,120 | 7 | 353 | 3 |
| 151,691 | 7 | 385 | 7 | 130,008 | 139,524 | 139,524 | 7 | 355 | 5 |
| 174,960 | 9 | 383 | 7 | 174,960 | 174,960 | 174,960 | 9 | 353 | 5 |
 This will be a Common Year following a Leap Year (CF).
This will be a Common Year following a Leap Year (CF).
Adding the numbers gives a total of 2457 648d 359 284p. The number of parts exceeds a week, so we subtract 181 440p and add 7d: 2457 655d 177 844p. When we examine the table for the excess of parts, we are over the threshold at 174 960p, which gives us a year 9/353/5, a year that begins on a Monday (7+2), is 353 days long, and will be followed by a year (5778) that begins on a Thursday.
Thus, for the final Julian Day Number, we add 9d for Monday and 1d for 1 Tišrî to our total of days: JD 2457 665d. This turns out to be 3 October 2016. This is the same day as 1 Muḥarram 1438 AH, 3 October 2016, the New Year on the Islamic Calendar. As the Islamic Year moves through the seasons, we always come to a period where the Jewish and Islamic New Years coincide.

| Results of all the Deḫiyyôth, 5778 | |||||||||
|---|---|---|---|---|---|---|---|---|---|
| L p | d | d/y | f | CF p | CB p | C p | d | d/y | f |
| 0 | 2 | 383 | 7 | 0 | 0 | 0 | 2 | 353 | 5 |
| 22,091 | 2 | 385 | 2 | 9,924 | 9,924 | 9,924 | 2 | 355 | 7 |
| 45,360 | 3 | 384 | 2 | 42,709 | 42,709 | 45,360 | 3 | 354 | 7 |
| 71,280 | 5 | 383 | 3 | 61,764 | 61,764 | 61,764 | 5 | 354 | 2 |
| 90,335 | 5 | 385 | 5 | 113,604 | 113,604 | 113,604 | 5 | 355 | 3 |
| 123,120 | 7 | 383 | 5 | 123,120 | 123,120 | 123,120 | 7 | 353 | 3 |
| 151,691 | 7 | 385 | 7 | 130,008 | 139,524 | 139,524 | 7 | 355 | 5 |
| 174,960 | 9 | 383 | 7 | 174,960 | 174,960 | 174,960 | 9 | 353 | 5 |
 This will be a Common Year (C).
This will be a Common Year (C).
Adding the numbers gives a total of 2458,005d 291,040p. The number of parts exceeds a week, so we subtract 181 440p and add 7d: 2458,012d 109,600p. When we examine the table for the excess of parts, we are over the threshold at 61,764p, which gives us a year 5/354/2, a year that begins on a Thursday (5), is 354 days long, and will be followed by a year (5779) that begins on a Monday (2).
Thus, for the final Julian Day Number, we add 5d for Thursday and 1d for 1 Tišrî to our total of days: JD 2458 018d. This turns out to be 21 September 2017. This is 1 day before 1 Muḥarram 1439 AH, 22 September 2017, the New Year on the Islamic Calendar.

| Results of all the Deḫiyyôth, 5779 | |||||||||
|---|---|---|---|---|---|---|---|---|---|
| L p | d | d/y | f | CF p | CB p | C p | d | d/y | f |
| 0 | 2 | 383 | 7 | 0 | 0 | 0 | 2 | 353 | 5 |
| 22,091 | 2 | 385 | 2 | 9,924 | 9,924 | 9,924 | 2 | 355 | 7 |
| 45,360 | 3 | 384 | 2 | 42,709 | 42,709 | 45,360 | 3 | 354 | 7 |
| 71,280 | 5 | 383 | 3 | 61,764 | 61,764 | 61,764 | 5 | 354 | 2 |
| 90,335 | 5 | 385 | 5 | 113,604 | 113,604 | 113,604 | 5 | 355 | 3 |
| 123,120 | 7 | 383 | 5 | 123,120 | 123,120 | 123,120 | 7 | 353 | 3 |
| 151,691 | 7 | 385 | 7 | 130,008 | 139,524 | 139,524 | 7 | 355 | 5 |
| 174,960 | 9 | 383 | 7 | 174,960 | 174,960 | 174,960 | 9 | 353 | 5 |
 This will be a Leap Year (L).
This will be a Leap Year (L).
Adding the numbers gives a total of 2458,362d 183,516p. The number of parts exceeds a week, so we subtract 181 440p and add 7d: 2458,369d 2,076p. When we examine the table for the excess of parts, we are under the threshold at 22,091p, which gives us a year 2/383/7, a year that begins on a Monday (2), is 383 days long, and will be followed by a year (5780) that begins on a Saturday (7).
Thus, for the final Julian Day Number, we add 2d for Monday and 1d for 1 Tišrî to our total of days: JD 2458 372d. This turns out to be 10 September 2018. This will be 2 days before 1 Muḥarram 1440 AH, 12 September 2018, the New Year on the Islamic Calendar. This coincidence of the Jewish and Islamic New Year will end after the Jewish calendar adds a month in AM 5779.
There is an error here that I have not been able to track down. The year 5779 should be a 2/385/2 year, but I don't show enough parts to reach the threshold for it, nowhere near. 5780 begins on a Monday, with 385 days elapsed. This is not a problem with subsequent years, so there is some kind of mistake in my table here. I am perplexed.

| Results of all the Deḫiyyôth, 5780 | |||||||||
|---|---|---|---|---|---|---|---|---|---|
| L p | d | d/y | f | CF p | CB p | C p | d | d/y | f |
| 0 | 2 | 383 | 7 | 0 | 0 | 0 | 2 | 353 | 5 |
| 22,091 | 2 | 385 | 2 | 9,924 | 9,924 | 9,924 | 2 | 355 | 7 |
| 45,360 | 3 | 384 | 2 | 42,709 | 42,709 | 45,360 | 3 | 354 | 7 |
| 71,280 | 5 | 383 | 3 | 61,764 | 61,764 | 61,764 | 5 | 354 | 2 |
| 90,335 | 5 | 385 | 5 | 113,604 | 113,604 | 113,604 | 5 | 355 | 3 |
| 123,120 | 7 | 383 | 5 | 123,120 | 123,120 | 123,120 | 7 | 353 | 3 |
| 151,691 | 7 | 385 | 7 | 130,008 | 139,524 | 139,524 | 7 | 355 | 5 |
| 174,960 | 9 | 383 | 7 | 174,960 | 174,960 | 174,960 | 9 | 353 | 5 |
 This will be a Common Year following a Leap Year (CF).
This will be a Common Year following a Leap Year (CF).
Adding the numbers gives a total of 2458,740d 375,665p. The number of parts exceeds two weeks, so we subtract 362 880p and add 14d: 2458,754d 12,785p. When we examine the table for the excess of parts, we are under the threshold at 9,9241p, which gives us a year 2/355/7, a year that begins on a Monday (2), is 355 days long, and will be followed by a year (5781) that begins on a Saturday (7).
Thus, for the final Julian Day Number, we add 2d for Monday and 1d for 1 Tišrî to our total of days: JD 2458 757d. This turns out to be 30 September 2019. This will be 29 days after 1 Muḥarram 1441 AH, 1 September 2019, JD 2458 728d, the New Year on the Islamic Calendar. This separation of the Jewish and Islamic New Year resulted from year 5779 adding a month.

| Results of all the Deḫiyyôth, 5781 | |||||||||
|---|---|---|---|---|---|---|---|---|---|
| L p | d | d/y | f | CF p | CB p | C p | d | d/y | f |
| 0 | 2 | 383 | 7 | 0 | 0 | 0 | 2 | 353 | 5 |
| 22,091 | 2 | 385 | 2 | 9,924 | 9,924 | 9,924 | 2 | 355 | 7 |
| 45,360 | 3 | 384 | 2 | 42,709 | 42,709 | 45,360 | 3 | 354 | 7 |
| 71,280 | 5 | 383 | 3 | 61,764 | 61,764 | 61,764 | 5 | 354 | 2 |
| 90,335 | 5 | 385 | 5 | 113,604 | 113,604 | 113,604 | 5 | 355 | 3 |
| 123,120 | 7 | 383 | 5 | 123,120 | 123,120 | 123,120 | 7 | 353 | 3 |
| 151,691 | 7 | 385 | 7 | 130,008 | 139,524 | 139,524 | 7 | 355 | 5 |
| 174,960 | 9 | 383 | 7 | 174,960 | 174,960 | 174,960 | 9 | 353 | 5 |
 This will be a Common Year (C).
This will be a Common Year (C).
Adding the numbers gives a total of 2459,097d 307,421p. The number of parts exceeds one week, so we subtract 181 440p and add 7d: 2459,104d 125,981p. When we examine the table for the excess of parts, we are under the threshold at 123,120p, which gives us a year 7/353/3, a year that begins on a Saturday (7), is 353 days long, and will be followed by a year (5782) that begins on a Tuesday (3).
Thus, for the final Julian Day Number, we add 7d for Saturday and 1d for 1 Tišrî to our total of days: JD 2459 112d. This turns out to be 19 September 2020. This will be 30 days after 1 Muḥarram 1442 AH, 20 August 2020, JD 2459 082d, the New Year on the Islamic Calendar. This separation of the Jewish and Islamic New Year resulted from year 5779 adding a month.
| Results of all the Deḫiyyôth, 5782 | |||||||||
|---|---|---|---|---|---|---|---|---|---|
| L p | d | d/y | f | CF p | CB p | C p | d | d/y | f |
| 0 | 2 | 383 | 7 | 0 | 0 | 0 | 2 | 353 | 5 |
| 22,091 | 2 | 385 | 2 | 9,924 | 9,924 | 9,924 | 2 | 355 | 7 |
| 45,360 | 3 | 384 | 2 | 42,709 | 42,709 | 45,360 | 3 | 354 | 7 |
| 71,280 | 5 | 383 | 3 | 61,764 | 61,764 | 61,764 | 5 | 354 | 2 |
| 90,335 | 5 | 385 | 5 | 113,604 | 113,604 | 113,604 | 5 | 355 | 3 |
| 123,120 | 7 | 383 | 5 | 123,120 | 123,120 | 123,120 | 7 | 353 | 3 |
| 151,691 | 7 | 385 | 7 | 130,008 | 139,524 | 139,524 | 7 | 355 | 5 |
| 174,960 | 9 | 383 | 7 | 174,960 | 174,960 | 174,960 | 9 | 353 | 5 |
Adding the numbers gives a total of 2459,454d 239,177p. The number of parts exceeds one week,  so we subtract 181 440p and add 7d: 2459,461d 57,737p. When we examine the table for the excess of parts, we are under the threshold at 45,360p, which gives us a year 3/384/2, a year that begins on a Tuesday (3), is 384 days long, and will be followed by a year (5783) that begins on a Monday (2).
so we subtract 181 440p and add 7d: 2459,461d 57,737p. When we examine the table for the excess of parts, we are under the threshold at 45,360p, which gives us a year 3/384/2, a year that begins on a Tuesday (3), is 384 days long, and will be followed by a year (5783) that begins on a Monday (2).
Thus, for the final Julian Day Number, we add 3d for Tuesday and 1d for 1 Tišrî to our total of days: JD 2459 465d. This turns out to be 7 September 2021. This will be 28 days after 1 Muḥarram 1443 AH, 10 August 2021, JD 2459 437d, the New Year on the Islamic Calendar.
| Results of all the Deḫiyyôth, 5783 | |||||||||
|---|---|---|---|---|---|---|---|---|---|
| L p | d | d/y | f | CF p | CB p | C p | d | d/y | f |
| 0 | 2 | 383 | 7 | 0 | 0 | 0 | 2 | 353 | 5 |
| 22,091 | 2 | 385 | 2 | 9,924 | 9,924 | 9,924 | 2 | 355 | 7 |
| 45,360 | 3 | 384 | 2 | 42,709 | 42,709 | 45,360 | 3 | 354 | 7 |
| 71,280 | 5 | 383 | 3 | 61,764 | 61,764 | 61,764 | 5 | 354 | 2 |
| 90,335 | 5 | 385 | 5 | 113,604 | 113,604 | 113,604 | 5 | 355 | 3 |
| 123,120 | 7 | 383 | 5 | 123,120 | 123,120 | 123,120 | 7 | 353 | 3 |
| 151,691 | 7 | 385 | 7 | 130,008 | 139,524 | 139,524 | 7 | 355 | 5 |
| 174,960 | 9 | 383 | 7 | 174,960 | 174,960 | 174,960 | 9 | 353 | 5 |
Adding the numbers gives a total of 2459,839d 200,606p. The number of parts exceeds one week,  so we subtract 181 440p and add 7d: 2459,846d 19,166p. When we examine the table for the excess of parts, we are under the threshold at 42,709p, which gives us a year 2/355/7, a year that begins on a Monday (2), is 355 days long, and will be followed by a year (5784) that begins on a Saturday (7).
so we subtract 181 440p and add 7d: 2459,846d 19,166p. When we examine the table for the excess of parts, we are under the threshold at 42,709p, which gives us a year 2/355/7, a year that begins on a Monday (2), is 355 days long, and will be followed by a year (5784) that begins on a Saturday (7).
Thus, for the final Julian Day Number, we add 2d for Monday and 1d for 1 Tišrî to our total of days: JD 2459 849d. This turns out to be 26 September 2022. This will be 58 days after 1 Muḥarram 1444 AH, 30 July 2022, JD 2459 791d, the New Year on the Islamic Calendar.
| Results of all the Deḫiyyôth, 5784 | |||||||||
|---|---|---|---|---|---|---|---|---|---|
| L p | d | d/y | f | CF p | CB p | C p | d | d/y | f |
| 0 | 2 | 383 | 7 | 0 | 0 | 0 | 2 | 353 | 5 |
| 22,091 | 2 | 385 | 2 | 9,924 | 9,924 | 9,924 | 2 | 355 | 7 |
| 45,360 | 3 | 384 | 2 | 42,709 | 42,709 | 45,360 | 3 | 354 | 7 |
| 71,280 | 5 | 383 | 3 | 61,764 | 61,764 | 61,764 | 5 | 354 | 2 |
| 90,335 | 5 | 385 | 5 | 113,604 | 113,604 | 113,604 | 5 | 355 | 3 |
| 123,120 | 7 | 383 | 5 | 123,120 | 123,120 | 123,120 | 7 | 353 | 3 |
| 151,691 | 7 | 385 | 7 | 130,008 | 139,524 | 139,524 | 7 | 355 | 5 |
| 174,960 | 9 | 383 | 7 | 174,960 | 174,960 | 174,960 | 9 | 353 | 5 |
Adding the numbers gives a total of 2460,189d 323,802p. The number of parts exceeds one week,  so we subtract 181 440p and add 7d: 2460,196d 142,362p. When we examine the table for the excess of parts, we are under the threshold at 151,691p, which gives us a year 7/383/5, a year that begins on a Saturday (7), is 383 days long, and will be followed by a year (5785) that begins on a Thursday (5).
so we subtract 181 440p and add 7d: 2460,196d 142,362p. When we examine the table for the excess of parts, we are under the threshold at 151,691p, which gives us a year 7/383/5, a year that begins on a Saturday (7), is 383 days long, and will be followed by a year (5785) that begins on a Thursday (5).
Thus, for the final Julian Day Number, we add 7d for Monday and 1d for 1 Tišrî to our total of days: JD 2460 204d. This turns out to be 16 September 2023. This will be 59 days after 1 Muḥarram 1445 AH, 19 July 2023, JD 2460 145d, the New Year on the Islamic Calendar.

 The Jewish and Moslem Calendars with the Era of Nabonassar
The Jewish and Moslem Calendars with the Era of Nabonassar
Philosophy of Religion, Calendars
Judaea of the Maccabees and Herodians
The State of Israel, 1948-present
Philosophy of History, Calendars
Before modern geology, the only estimates of the age of the world were religious. In India, we have vast cycles of times in an essentially eternal universe. In the West, estimates for a temporally finite universe were based on revelation. Judaism and Christianity had substantial material to work with in the chronology and counts of generations in the Bible. This material, however, was ambiguous, and we end up with a wide spread of estimates, over a range of almost two thousand years.

The Jewish Eras of the World

| 6498 Cycle until 622 AD | 5877 BC |
| Byzantine Era, as of 988 AD | 5509 BC |
| Maximus the Confessor (c.580- 662) | 5493 BC |
| William Hales (1747-1831), A New Analysis of Chronology | 5411 BC |
| Scaliger's Julian Period | 4713 BC |
| 6498 Cycle until 1990 AD | 4509 BC |
   Seder Olam Zûṭâ, Small Chronicle of the World, 1121 AD | 4359 BC |
| Eastern Jews, according to ʾAbu l-Farangi | 4220 BC |
| Western Jews, according to Riccioli | 4184 BC |
| Chinese Jews, according to Brotier | 4079 BC |
| Moses Maimonides, Universal History | 4058 BC |
| Bishop James Ussher (1581-1656) | 4004 BC |
| Sir Isaac Newton (1643-1727) | c.4000 BC |
| Johannes Kepler (1571-1630) | 3992 BC |
| The Venerable Bede (c.672-735) | 3952 BC |
| Joseph Justus Scaliger (1540-1609) | 3949 BC |
| John Lightfoot (1602-1675) | 3929 BC |
| David Ganz, Chronology | 3761 BC |
| accepted Jewish Annō Mundi | 3760 BC |
| Rabbi Gersom, Playfair | 3754 BC |
   Seder Olam Rabba, Great Chronicle of the World, 130 AD | 3751 BC |
| Rabbi Habsom, Universal History | 3740 BC |
| Rabbi Nosen, Universal History | 3734 BC |
| Rabbi Hillel, circa 358 AD | 3700 BC |
| Rabbi Zachuth, Universal History | 3671 BC |
| Rabbi Lipman, Universal History | 3616 BC |
When I was in High School, I used to ask Jewish friends, often when Rōʾš Haššānâh, 
 , would come around, what it is that the era of the Jewish Calendar actually dated. They did not know. I had to read Isaac Asimov to discover that it was the Creation. The era was an Annō Mundi (AM), an "in the year of the world," date. The famliar Jewish Era goes back to 3760 BC, but, as in Christianity, this has not been the only estimate of the age of the world in the history of Judaism.
, would come around, what it is that the era of the Jewish Calendar actually dated. They did not know. I had to read Isaac Asimov to discover that it was the Creation. The era was an Annō Mundi (AM), an "in the year of the world," date. The famliar Jewish Era goes back to 3760 BC, but, as in Christianity, this has not been the only estimate of the age of the world in the history of Judaism.
My source on the variety of Jewish dates of Creation, and the main reason why this treatment is called "Jewish Eras of the World," was a book I found while digging through the main library at the University of Texas: Modern Judaism: or a brief account of the opinions, traditions, rites, and ceremonies of the Jews in modern times, by John Allen (1771-1843) [2nd Edition, R.B. Seeley and W. Burnside, London, 1830, pp.366-367]. Since this was a book published in 1830, the "Modern" in the title now looks a little incongruous. But it is nice to see this list from a relatively naive source, i.e. one unaware of the subsequent history of geology and Darwinism.
Allen was unaware of any certain source of the era, from 3760 BC, that had actually already become customary with the Jewish calendar. However, he had information of suggested eras from many sources in both Jewish and Christian scholarship. Almost all of the dates shown here are therefore from his book. The "Universal History" referenced by Allen may be An Universal History: From the Earliest Accounts to the Present Time, by George Sale, George Psalmanazar, Archibald Bower, George Shelvocke, John Campbell, John Swinton [C. Bathurst, London, 1759]. "Rabbi Hillel" seems to mean the Palestinian Patriarch who can be credited with instituting, if nothing else, the 19 year cycle in the Jewish Calendar.
In the Christian context, the most famous estimate of Creation is certainly that of the Irish Archbishop James Ussher, who thought that the first day of the World was 23 October 4004 BC on the Julian Proleptic Calendar, a day reckoned, however, to have begun (in the Babylonian, Jewish, and Islamic fashion) the previous sunset. Since this date was used in many English editions of the Bible in the 19th century, many people, like William Jennings Bryan (1860-1925), were left with the impression that this was the universally agreed result of Biblical research. Thus, Byran would reference it in his Creationist prosecution in the Scopes "Monkey Trial" of 1925. However, there were many Biblical estimates of the age of the world in Ussher's own 17th century, and the ones that had been used the longest originated with Byzantine historians as far back as the beginning of the 7th century.
 Indeed, Mediaeval historians writing in Greek came to all use the "Byzantine" Era. This did not catch on in the West, and the Era that now tends to get used in chronology is the "Julian Period" of Joseph Justus Scaliger (1540-1609), whose benchmark is 4713 BC. Scalinger arrived at this by looking for the date when various calendar cycles, including the 15 year Roman Indiction cycle, would all have stood at "Year 1." This benchmark is what is used for Julian Dates. Its convenience is that, like the Byzantine Era, it antedates all historical time, which cannot be pushed back earlier than the Archaic Period of Egypt. Nevertheless, he seems to have dated the actual Creation to 3949 BC.
Indeed, Mediaeval historians writing in Greek came to all use the "Byzantine" Era. This did not catch on in the West, and the Era that now tends to get used in chronology is the "Julian Period" of Joseph Justus Scaliger (1540-1609), whose benchmark is 4713 BC. Scalinger arrived at this by looking for the date when various calendar cycles, including the 15 year Roman Indiction cycle, would all have stood at "Year 1." This benchmark is what is used for Julian Dates. Its convenience is that, like the Byzantine Era, it antedates all historical time, which cannot be pushed back earlier than the Archaic Period of Egypt. Nevertheless, he seems to have dated the actual Creation to 3949 BC.
A curious featue of the Mundane Eras is that we get two groups of Jewish dates and two of Christian, with the very oldest the first Christian group and the very youngest the second Jewish group.
| 1368 y |  = 16920 m | ||||
|---|---|---|---|---|---|
| 235 m | x 72 | ||||
| 47 | x 5 | x 6 | x 4 | x 3 | |
| x 30 y | x 12 m | ||||
| 1410 y | |||||
I am not aware of Muslim scholars trying to formulate Eras of the World. The Qurʾân does not contain the chronologies and genealogies that the Bible does, which is the common source for both Jewish and Christian reckoning. My impression is that Islâmic scholars tend to be uninterested in pre-Islâmic history.
 The Jewish and Moslem Calendars with the Era of Nabonassar
The Jewish and Moslem Calendars with the Era of Nabonassar
Philosophy of Religion, Calendars
Philosophy of History, Calendars
There seems little call to have a modern luni-solar calendar, which keeps track of both the seasons and the Moon. Surviving luni-solar calendars, the Jewish and the Chinese, are now used mainly for ritual purposes. The phenomenology of the But the principles are simple enough. The Full Moon rises at sunset. Waxing moons always rise before sunset; and waning moons always rise afterwards. At local apparent noon, the Sun is on the Meridian, the imaginary line in the sky that passes from due South to due North through the Zenith, the point directly overhead. "Local apparent noon" means the moment when the visible Sun is actually on the Meridian. This is when sailors take the Noon Sighting.
The Full Moon is on the Meridian at local apparent Midnight, i.e. twelve hours after Noon [note]. The First Quarter Moon is on the Meridian at sunset; and the Third Quarter Moon is on the Meridian at sunrise. At sunset, the waxing Crescent Moon is somewhere between the Meridian and the Western horizon. At sunrise, the waning Crescent Moon is somewhere between the Meridian and the Eastern horizon. A very Young Cresent or a very Old Cresent are close to their respective horizons at sunset or sunrise. A New Moon, of course, rises and sets with the Sun and is invisible -- unless a solar eclipse occurs, when the Moon betrays its presence by blocking the light of the Sun. Because the Full Moon is always opposite the Sun on the ecliptic, Full Moons in Winter are high in the sky, where the Sun would be in the Summer; and Full Moons in Summer are low in the sky, where the Sun would be in Winter. Thus, a Winter Full Moon on snow can light up the landscape considerably, giving an impression almost of daylight.
Since the First Quarter Moon is on the Meridian at sunset, and the Full Moon rises as sunset, and that will be a quarter month, or 7.4 days later, and the horizon is 90o from the Meridian, the Moon moved about 12.2o a day from one to the other. We might wonder about how this works when the Synodic Month, the period (περίοδος) of the Moon in relation to the Sun, 29.5 days, is different from the Sidereal Month, the period of the Moon in relation to the Stars. The Sidereal Month is only 27.3 days long. That means that the Moon must move against the Stars at a rate of 13.2 degrees a day (360/27.3). This differs from the Synodic Month, because the Sun is a moving target. The Sun moves against the Stars at a rate of 0.99o a day. When the Moon returns to its position relative to the Stars, the Sun has advanced another 26.9o. The Moon needs to play catch-up. It takes another 2.2 days. In the frame of reference with respect to the Sun, the Moon moves 360o in 29.5 days, which comes to 12.2o per day. This makes it look like the Moon moves slower in relation to the Sun than it does in relation to the Stars. And it does. The Sun lengthens the month by advancing ahead, but shortens the month by advancing from behind. I find this a little confusing.
For the future, there is at least one conceivable reason why a luni-solar calendar might eventually be desired: When there are human colonies on the Moon, it will be of rather more practical concern than it is on Earth what phase the Moon is in, since that determines whether it is day or night outside. For lunar inhabitants, this may or may not turn out to be of importance in their lives (since the surface conditions may not be of that much significance, except for those required to go outside), but they might like an intuitive way of keeping track of it anyway.
Since the rules for determining the actual date are complicated in both the Jewish and Chinese calendars, something simpler might be in order. The problem of any luni-solar calendar is its dual purpose. Keeping track of the Moon requires adjustment of days. Keeping track of the Sun requires adjustment of months. The basic day pattern for the year can be borrowed from the practice of the Jewish and Islâmic calendars, i.e. an alternation of 30 and 29 day months. The 19 year cycle then adds 7 months every 19 years. As considered for the Babylonian calendar above, if six of those months are 30 days each, and the 7th (the Ululu II that occurs only once every 19 years) is 29 days, this averages out to exactly 365 days per year: (19 x ((6 x 30) + (6 x 29)) + (6 x 30) + 29)/19 = 365. Intercalary days must then be added to this to approximate the Metonic year.
The easiest rule for adding days to a year is the Julian intercalation, i.e. an extra day every 4 years. This is familiar and very easy to keep track of. It is also more accurate for the Metonic year than for the tropical year -- off a day in 307 years rather than the day in 128 years that the Julian calendar errs against the seasons. This also gives us the simplest correction to use for the Julian intercalation: Century years evenly divisible by 300 would not be leap years for our luni-solar calendar = 365 +1/4 -1/300. This gives a year of 365.246 days [where "6" is a repeating decimal]. That takes care of the Moon. The remaining problem, however, is that the Metonic year is not accurate enough for the Sun. It is off a day in 219 years -- more accurate than the Julian calendar (off a day in 128 years), but not by much. The 365 +1/4 -1/300 day year is off a day in 224 years. Retaining the 19 year cycle means that it must occasionally be adjusted. It runs slow, and so the dates fall later in the year over time. The way to adjust it is to periodically delay one of the intercalations of months.
In a 19 year cycle, one year starts the latest. This will follow the intercalary year that itself starts the latest. When the latest year is starting too late, the intercalation in the previous year can be delayed into the following year, which means that the year starting the latest suddenly becomes the year starting the earliest. As it happens this can be conveniently done after exactly 18 of the 19 year cycles, i.e. every 342 years. After every delay has been done 19 times, i.e. once for every year in the cycle, we get a larger cycle in which the pattern of delays will occur again -- 342 x 19 = 6498 years. After a complete 6498 year cycle, what ends up happening is that one of the 29 day months disappears; it has in effect been delayed out of existence. Now, 365 +1/4 -1/300 -29/6498 gives us an average solar year of 365.2422038 days. This only off a day against the tropical year in over 200,000 years -- accurate far beyond the limits of certainty, just as the 6498 year cycle itself is longer than human history -- making it comparable to the Eras of the World contemplated in Judaism and Christianity.
Such a calendar serves the purpose. Four things have to be kept track of: (1) the leap day every 4 years, (2) the loss of a leap day every 300 years, (3)

A Modern Luni-Solar Calendar


 On the other hand, the tides are strongly affected by the phases of the moon, and sailors and fishermen may keep in mind that the tides are the strongest at the New and Full Moons. At the same time, as ancient people were certainly aware, the lunar month matched up closely with the menses of women. The sort of feminists who wish to valorize the feminine certainly highlight the affinity of the feminine to the Moon; but I have never met any women who actually paid any attention to lunar calendar months -- although female calendar buffs cannot fail but be aware of them.
On the other hand, the tides are strongly affected by the phases of the moon, and sailors and fishermen may keep in mind that the tides are the strongest at the New and Full Moons. At the same time, as ancient people were certainly aware, the lunar month matched up closely with the menses of women. The sort of feminists who wish to valorize the feminine certainly highlight the affinity of the feminine to the Moon; but I have never met any women who actually paid any attention to lunar calendar months -- although female calendar buffs cannot fail but be aware of them.
 lunar month is something not familiar to everyone, despite the universal possibility of paying direct attention to it. Thus, the fact that the time of moonrise is directly tied to the phase of the moon is something of which not everyone seems to be aware. Years ago I read a fantasy writer who seemed to be under the impression that the moon rose at the same time every night, and it could then be exhibiting any phase. One wonders how often he had ever actually looked at the moon.
lunar month is something not familiar to everyone, despite the universal possibility of paying direct attention to it. Thus, the fact that the time of moonrise is directly tied to the phase of the moon is something of which not everyone seems to be aware. Years ago I read a fantasy writer who seemed to be under the impression that the moon rose at the same time every night, and it could then be exhibiting any phase. One wonders how often he had ever actually looked at the moon.

 Adding or subtracting days, as the Julian or Gregorian calendars do, to adjust the seasons will not work, since this will ruin correspondence to the Moon. The best convenient rule for the Sun is thus still the Babylonian 19 year cycle. The continued fraction for the number of synodic months per year (12.368267058) can be seen at right. Adding 123 months every 334 years would be very cumbersome to keep track of. Another virtue of the 19 year cycle is that is can be used to keep track of the Moon also. There are 235 months per 19 year cycle, and this averages out to 365.2467463 days/year. This means that the number of days per year in our calendar will track the Moon if it approximates the length of this "Metonic" year -- i.e. fitting the 235 lunar months rather than the actual 19 solar (tropical, i.e. tracking the seasons) years.
Adding or subtracting days, as the Julian or Gregorian calendars do, to adjust the seasons will not work, since this will ruin correspondence to the Moon. The best convenient rule for the Sun is thus still the Babylonian 19 year cycle. The continued fraction for the number of synodic months per year (12.368267058) can be seen at right. Adding 123 months every 334 years would be very cumbersome to keep track of. Another virtue of the 19 year cycle is that is can be used to keep track of the Moon also. There are 235 months per 19 year cycle, and this averages out to 365.2467463 days/year. This means that the number of days per year in our calendar will track the Moon if it approximates the length of this "Metonic" year -- i.e. fitting the 235 lunar months rather than the actual 19 solar (tropical, i.e. tracking the seasons) years.
 Against the Metonic year, that is only off a day in 12,555 years, at least four times as accurate as the Gregorian calendar, and even more accurate for the Moon than the purely lunar Islâmic calendar.
Against the Metonic year, that is only off a day in 12,555 years, at least four times as accurate as the Gregorian calendar, and even more accurate for the Moon than the purely lunar Islâmic calendar.
| 1368 y |  = 16920 m | ||||
|---|---|---|---|---|---|
| 235 m | x 72 | ||||
| 47 | x 5 | x 6 | x 4 | x 3 | |
| x 30 y | x 12 m | ||||
| 1410 y | |||||
Another way to do this calendar, however, is set aside the Julian intercalation and the 300 year correction and to piggyback our reckoning onto the Gregorian calendar. This makes it easier to determine actual dates, Gregorian dates, for luni-solar reckoning. The Gregorian year is 365 +1/4 -3/400 = 365.2425 days. This is off one day in 3320 years against the seasons and one day in 220 years against the Metonic year. Exactly 12 of the 19 year cycles equals 228 years. If we figure a day correction after that span we get, 365 +1/4 -3/400 +1/228 = 365.246886. This is off just a day in 7161 years against the Metonic year. This is quite accurate enough for our purposes, indeed more than twice as accurate as the Gregorian year is for the Sun. If we figure in the 342 year corrections of the 19 year cycle, we get 365 +1/4 -3/400 +1/228 -29/6498 = 365.2424231. This is off a day against the Sun in 4459 years, more accurate than the Gregorian calendar itself, but in the same order of magnitude.
| 1368 y | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| 16920 m | |||||||||||
| 684 y | 684 y | ||||||||||
| 8460 m | 8460 m | ||||||||||
| 342 y | 342 y | 342 y | 342 y | ||||||||
| 4230 m | 4230 m | 4230 m | 4230 m | ||||||||
| 228 y | 228 y | 228 y | 228 y | 228 y | 228 y | ||||||
| 2820 m | 2820 m | 2820 m | 2820 m | 2820 m | 2820 m | ||||||
A 228 year cycle also turns out to be quite convenient when we realize that three of them are equal to two 342 year cycles: 3 x 228 = 2 x 342 = 684 years. This is also, by a nice coincidence, actually half of the 1368 years that has been noted as the period in which the cycles of the Jewish and Islâmic calendars are commensurable -- 1368 years also being the span between the beginning of the Era of Nabonassar (747 BC) and the beginning of the Islâmic Hegira Era (622 AD). A further 1368 years brings us down to our time, to 1990 AD.
In constructing the Gregorian dates for our luni-solar calendar, we operate on the principle that the year, using the Babylonian New Year, should start on or after the Vernal Equinox. This is defined as March 21st for the Gregorian calendar, but it usually occurs on March 20th (Universal Time). As it happens, the calendar can be conveniently designed so that the earliest New Year in each 19 year cycle just ranges from March 20th to March 22nd. The latest New Year then ranges from April 17th to April 19th. Actual dates can then be constructed using a simple rule from the Gregorian Easter reckoning: Each year, the lunar dates occur 11 days earlier. Thus, a New Year one year on 4/15 will occur on 4/4 the next year.
 AN, Era of AN, Era ofNabonassar | ||||||||
|---|---|---|---|---|---|---|---|---|
| 1 | 229 | 343 | 457 | 685 | 913 | 1027 | 1141 | |
| BC | AD | |||||||
| 747 | 519 | 405 | 291 | 63 | 166 | 280 | 394 | |
| 0 | 3/27* | 3/28* | 3/28* | 3/29* | 3/30* | 3/31* | 3/31 | 4/1 |
| 1 | 4/15 | 4/16 | 4/16 | 4/17 | 4/18> | 4/19> | 3/20<§ | 3/21<§ |
| 2 | 4/4 | 4/5 | 4/5 | 4/6 | 4/7 | 4/8 | 4/7 | 4/8 |
| 3 | 3/24* | 3/25* | 3/25* | 3/26* | 3/27* | 3/28* | 3/27* | 3/28* |
| 4 | 4/12 | 4/13 | 4/13 | 4/14 | 4/15 | 4/16 | 4/15 | 4/16 |
| 5 | 4/1 | 4/2 | 4/2 | 4/3 | 4/4 | 4/5 | 4/4 | 4/5 |
| 6 | 3/21<§ | 3/22<§ | 3/22* | 3/23* | 3/24* | 3/25* | 3/24* | 3/25* |
| 7 | 4/8 | 4/9 | 4/10 | 4/11 | 4/12 | 4/13 | 4/12 | 4/13 |
| 8 | 3/28* | 3/29* | 3/30* | 3/31* | 4/1 | 4/2 | 4/1 | 4/2 |
| 9 | 4/16 | 4/17 | 4/18> | 4/19> | 3/21<§ | 3/22<§ | 3/21* | 3/22* |
| 10 | 4/5 | 4/6 | 4/7 | 4/8 | 4/8 | 4/9 | 4/9 | 4/10 |
| 11 | 3/25* | 3/26* | 3/27* | 3/28* | 3/28* | 3/29* | 3/29* | 3/30* |
| 12 | 4/13 | 4/14 | 4/15 | 4/16 | 4/16 | 4/17 | 4/17> | 4/18> |
| 13 | 4/2 | 4/3 | 4/4 | 4/5 | 4/5 | 4/6 | 4/6 | 4/7 |
| 14 | 3/22* | 3/23* | 3/24* | 3/25* | 3/25* | 3/26* | 3/26* | 3/27* |
| 15 | 4/10 | 4/11 | 4/12 | 4/13 | 4/13 | 4/14 | 4/14 | 4/15 |
| 16 | 3/30* | 3/31* | 4/1 | 4/2 | 4/2 | 4/3 | 4/3 | 4/4 |
| 17 | 4/18> | 4/19> | 3/21<§ | 3/22<§ | 3/22* | 3/23* | 3/23* | 3/24* |
| 18 | 4/7 | 4/8 | 4/8 | 4/9 | 4/10 | 4/11 | 4/11 | 4/12 |
| 19 | 3/27* | 3/28* | 3/28* | 3/29* | 3/30* | 3/31* | 3/31 | 4/1 |
In the table at left, which covers the first 1368 years of the Era of Nabonassar, "<" marks the year with the earliest New Year, ">" marks the year with the latest New Year, "*" marks ordinary intercalary years, and "§" marks the intercalation of the 29 day Ululu II. The later, it will be observed, always occurs in the year with the earliest New Year. The column with the darker shade of purple background, beginning in 405 BC (or 343 AN), is the one in which the classic Babylonian 19 year cycle was fixed, where the intercalations are in the 3rd, 6th, 8th, 11th, 14th, 17th, and 19th years, with the Ululu II in the 17th year. The 9th year is the one with the latest New Year. The intercalation in the 8th year is the one that is next delayed, in 63 BC (or 685 AN).
| AN | ||||||||
|---|---|---|---|---|---|---|---|---|
| 1369 | 1597 | 1711 | 1825 | 2053 | 2281 | 2395 | 2509 | |
 AH, AH,Hejira Era | ||||||||
| 1 | 229 | 343 | 457 | 685 | 913 | 1027 | 1141 | |
| AD | ||||||||
| 622 | 850 | 964 | 1078 | 1306 | 1534 | 1648 | 1762 | |
| 0 | 4/2 | 4/3 | 4/3 | 4/4 | 4/5 | 4/6 | 4/6 | 4/7 |
| 1 | 3/22* | 3/23* | 3/23* | 3/24* | 3/25* | 3/26* | 3/26* | 3/27* |
| 2 | 4/10 | 4/11 | 4/11 | 4/12 | 4/13 | 4/14 | 4/14 | 4/15 |
| 3 | 3/30* | 3/31* | 3/31 | 4/1 | 4/2 | 4/3 | 4/3 | 4/4 |
| 4 | 4/18> | 4/19> | 3/20<§ | 3/21<§ | 3/22* | 3/23* | 3/23* | 3/24* |
| 5 | 4/7 | 4/8 | 4/7 | 4/8 | 4/10 | 4/11 | 4/11 | 4/12 |
| 6 | 3/27* | 3/28* | 3/27* | 3/28* | 3/30* | 3/31* | 3/31 | 4/1 |
| 7 | 4/15 | 4/16 | 4/15 | 4/16 | 4/18> | 4/19> | 3/20<§ | 3/21<§ |
| 8 | 4/4 | 4/5 | 4/4 | 4/5 | 4/7 | 4/8 | 4/7 | 4/8 |
| 9 | 3/24* | 3/25* | 3/24* | 3/25* | 3/27* | 3/28* | 3/27* | 3/28* |
| 10 | 4/12 | 4/13 | 4/12 | 4/13 | 4/15 | 4/16 | 4/15 | 4/16 |
| 11 | 4/1 | 4/2 | 4/1 | 4/2 | 4/4 | 4/5 | 4/4 | 4/5 |
| 12 | 3/21<§ | 3/22<§ | 3/21* | 3/22* | 3/24* | 3/25* | 3/24* | 3/25* |
| 13 | 4/8 | 4/9 | 4/9 | 4/10 | 4/12 | 4/13 | 4/12 | 4/13 |
| 14 | 3/28* | 3/29* | 3/29* | 3/30* | 4/1 | 4/2 | 4/1 | 4/2 |
| 15 | 4/16 | 4/17 | 4/17> | 4/18> | 3/21<§ | 3/22<§ | 3/21* | 3/22* |
| 16 | 4/5 | 4/6 | 4/6 | 4/7 | 4/9 | 4/9 | 4/9 | 4/10 |
| 17 | 3/25* | 3/26* | 3/26* | 3/27* | 3/28* | 3/29* | 3/29* | 3/30* |
| 18 | 4/13 | 4/14 | 4/14 | 4/15 | 4/16 | 4/17 | 4/17> | 4/18> |
| 19 | 4/2 | 4/3 | 4/3 | 4/4 | 4/5 | 4/6 | 4/6 | 4/7 |
Also important to note is that the zero year in the tables, although the benchmark, is prior to the actual first year of the calendar cycle. 622 AD, then is year 1, not year 0, in the cycle; and its luni-solar New Year will be on 3/22.
In each of these 1368 periods, there are four intercalation delays, the first at the beginning. There are six day corrections. The delay and the day correction occur at the same time twice in the period, at the beginning and at the 684 year point. This coincidence frequently result in a two day change, rather than just one. Where this occurs, we sometimes have the phenomenon of the extra day being taken back later. Thus, in the 11th year of the cycles in the Hegira Era table, we go from 4/2 to 4/4 to 4/5 but then back to 4/4 again. This counterintuitive sort of retrograde movement occurs because of the way our 29 day leap month jumps around from one period to another. A similar oddity turns up in the Gregorian Easter tables.
 AN, AN,Nabonassar | ||
|---|---|---|
| 2737 | 2965 | |
| AH | ||
| 1369 | 1597 | |
| AD | ||
| 1990 | 2218 | |
| early | late | |
| 0 | 4/8 | 4/9 |
| 1 | 3/28* | 3/29* |
| 2 | 4/16 | 4/17 |
| 3 | 4/5 | 4/6 |
| 4 | 3/25* | 3/26* |
| 5 | 4/13 | 4/14 |
| 6 | 4/2 | 4/3 |
| 7 | 3/22* | 3/23* |
| 8 | 4/10 | 4/11 |
| 9 | 3/30* | 3/31* |
| 10 | 4/18> | 4/19> |
| 11 | 4/7 | 4/8 |
| 12 | 3/27* | 3/28* |
| 13 | 4/15 | 4/16 |
| 14 | 4/4 | 4/5 |
| 15 | 3/24* | 3/25* |
| 16 | 4/12 | 4/13 |
| 17 | 4/1 | 4/2 |
| 18 | 3/21§< | 3/22§< |
| 19 | 4/8 | 4/9 |
Comparing the corrected and uncorrected cycles, it can be noted that all seven intercalations have by this point been delayed to the next year.
| corrected | uncorrected | |||
|---|---|---|---|---|
| 0 | 2736/2755 | 4/8 | 1989/2008 | 4/7* |
| 1 | 2737/2756 | 3/28* | 1990/2009 | 4/26 |
| 2 | 2738/2757 | 4/16 | 1991/2010 | 4/15 |
| 3 | 2739/2758 | 4/5 | 1992/2011 | 4/4* |
| 4 | 2740/2759 | 3/25* | 1993/2012 | 4/23 |
| 5 | 2741/2760 | 4/13 | 1994/2013 | 4/12 |
| 6 | 2742/2761 | 4/2 | 1995/2014 | 4/1* |
| 7 | 2743/2762 | 3/22* | 1996/2015 | 4/20 |
| 8 | 2744/2763 | 4/10 | 1997/2016 | 4/9* |
| 9 | 2745/2764 | 3/30* | 1998/2017 | 4/28> |
| 10 | 2746/2765 | 4/18> | 1999/2018 | 4/17 |
| 11 | 2747/2766 | 4/7 | 2000/2019 | 4/6* |
| 12 | 2748/2767 | 3/27* | 2001/2020 | 4/25 |
| 13 | 2749/2768 | 4/15 | 2002/2021 | 4/14 |
| 14 | 2750/2769 | 4/4 | 2003/2022 | 4/3* |
| 15 | 2751/2770 | 3/24* | 2004/2023 | 4/22 |
| 16 | 2752/2771 | 4/12 | 2005/2024 | 4/11 |
| 17 | 2753/2772 | 4/1 | 2006/2025 | 3/31§< |
| 18 | 2754/2773 | 3/21§< | 2007/2026 | 4/18 |
| 19 | 2755/2774 | 4/8 | 2008/2027 | 4/7* |
All of these dates are based on the Babylonian rule for the beginning of the Month: Not the New Moon, but the first day on which the Young Moon, a crescent, can be seen right after sunset. This is usually the second calendar day after the New Moon, though it must then be remembered that the Babylonian day (like the Jewish and Islâmic) begins at sunset of the day before. The Jewish month can begin with the New Moon, but usually it is delayed for ritual or other reasons into the range of the visible crescent. The Islâmic month, like the Babylonian, is supposed to begin with the crescent. If we want a luni-solar calendar based on the New Moon, all these dates must be advanced by a couple of days.
Without using the tables, the character of a year within the 6498 year cycle can be determined mathematically. If the remainder of the formula ((Y x 2393) + 1025)/6498, where Y is the year of the Era of Nabonassar (AN), runs from 0 to 2051, the year is a leap year with a 30 day leap month (*). If the remainder runs from 2052 to 2392, the year is a leap year with a 29 day leap month (§). Other remainders are for common years. 2393 is the number of leap years in the 6498 year cycle. This is one less than 7 times the number of 19 year cycles (342 x 7 = 2394). It can be determined that the 19 year period from 5130 to 5148 (counting 0-18 in the 19 year cycle) contains only 6 intercalations, and no 29 day month. By one reckoning (starting with 0 rather than 1), this is where the 2394th leap month disappears.

 The Jewish and Moslem Calendars with the Era of Nabonassar
The Jewish and Moslem Calendars with the Era of Nabonassar
Philosophy of Science, Calendars
"A.M." and "P.M.," which abbreviate ante meridian and post meridian, respectively, orient time to before and after Noon, whether this is Local Apparent Noon, Local Mean Noon (i.e. having calculated the average speed of the Sun, which otherwise varies with Season), Zone Mean Noon (e.g. Greenwich Mean Time minus 8 hours), or Mean Noon for a particular conventional time zone (e.g. Pacific Time). A 24 hour clock eliminates this complication, with Midnight as 00:00 hours and Noon as 12:00. This is reversed in the previous reckoning of Nautical or Astronomical Time, and the continuing Julian Date. There, Noon is 00:00 and Midnight is 12:00 hours. The calendar day begins at Noon.
Meteorologists and others seem to have adopted the strange convention that Noon is A.M. time and Midnight is P.M. Now, Midnight can reasonably be either A.M. or P.M., since it is equally 12 hours either way from Noon, but this choice and complication can be skipped just by calling it "Midnight." On the other hand, Noon cannot be "A.M." since it is the Sun on the Meridian itself, and so cannot be "before" the Meridian. Why this error is committed is something I can only imagine will be disclosed by inquiry to the people who commit it.
Return to Text
There is a completely separate Indian calendrical tradition. In the "Book of the Cattle Raid," in the Book of Virâṭa, in the Mahâbhârata, Duryodhana claims that the Pâṇḍavas have failed to keep their agreement to stay in exile for twelve years and in hiding for one. Bhiṣma reckons (47.1-5) that they have kept the agreement, and he mentions that the calendar adds an extra month every five years. A.L. Basham, in The Wonder that was India [1954, 1967, Rupa & Company, Calcutta, etc., 1981, 1989], states the calendar rule as adding an extra month every thirty months (p. 494). Sixty months is five years (5x12). That means two months in five years. Basham also says that this was done "as in Babylonia." But that is not true. The Babylonians added seven lunar months every nineteen years, which is often called the "Metonic" cycle (after the Greek astronomer Meton) and is still used by the Jewish calendar.
The economist Amartya Sen has a discussion of Indian calendars in his recent The Argumentative Indian, Writings on Indian History, Culture and Identity [Allen Lane, Penguin, 2005]. His essay, "India through its Calendars" [pp.317-333], however, is almost entirely about the Eras used in different calendars, not about the calendars themselves. One might be left not realizing that the same calendar can use different Eras, or that the same Era can be used by different calendars. The only actual calendar rule he mentions is that of the Mahâbhârata, though its meaning is clarified: "each year consists of twelve months of thirty days, with a thirteenth (leap) month added every five years" [p.325]. The use of thirty day months makes it plain that we are dealing with approximations to the solar year, but this rule produces a formidably poor approximation. If a thirty day month is added every five years, this averages out to a 366 day year, which would be off no less than three days in that period. Thus, a shorter intercalation would be needed, either 26 or 27 days, depending on one's estimation of the true length of the tropical year. Sen does mention that the mathematician Varâhamihra, in the sixth century AD, gave the length of the year as 365.25875 days [p.329]. This is significantly too long (over the 365.24220 days of the tropical year) and would be off a day in only 60 years. Such a value would be a discouraging glimpse into Indian astronomy, in that the Greeks had much better values much earlier, except that Varâhamihra's value was probably for the sidereal rather than the tropical year. Sen does mention the sidereal year (365.25636, or 365.25636042, days), the movement of the sun against to the stars rather than relative to the equinoxes, but he also doesn't say why he gives it. Taking Varâhamihra's value to be for the sidereal year, it is accurate to a day in 418 years.
Sen does not discuss how calendars were regulated given inaccurate rules like that of the Mahâbhârata or values for the sidereal year, which is otherwise not used for civil calendars. I was left with the impression that the calendars may actually have been regulated "as in Babylonia" in its historically earlier sense, i.e. with months inserted as needed, without any prior rule or calculation being applied. All this required was some established political or priestly authority with the recognized function of doing so. Since the moment of the Vernal Equinox can easily be observed from the kinds of observatories that were built in mediaeval India, the authorities need merely have inserted the extra month when the year otherwise would have begun before the Equinox.
A satisfactory treatment of the Indian calendar can now be found in The Oxford Companion to the Year, An exploration of calendar customs and time-reckoning [Bonnie Blackburn & Leofranc Holford-Strevens, Oxford U Press, 1999, 2003, pp.715-721].

A Modern Luni-Solar Calendar, Note


The Calendar in India

 Lunar Months, Lunar Months,now Solar Months | |
|---|---|
 |  March 22/21- March 22/21-April 20 |
 or, or, |  April 21- April 21-May 21 |
 |  May 22- May 22-June 21 |
 |  June 22- June 22-July 22 |
 |  July 23- July 23-August 22 |
 or, or, |  August 23- August 23-September 22 |
 or, or, |  September September23-October 22 |
 |  October 23- October 23-November 21 |
 or, or, |  November 22- November 22-December 21 |
 or, or, |  December 22- December 22-January 20 |
 |  January 21- January 21-February 19 |
 |  February 20- February 20-March 21/20 |
 is the Hindi version of
is the Hindi version of  . Elsewhere, where two names are given, they are both alternatives in Sanskrit.
. Elsewhere, where two names are given, they are both alternatives in Sanskrit.
The rule of the Mahâbhârata, so poor for a solar year, may have been intended, as in Basham's reading, to mean two lunar months in five years. For a lunar calendar adjusting to the solar year, the best approximations (by continued fractions) to the difference between twelve synodic months and the tropical year would be to add one month every three years, three every eight, four every eleven, seven every nineteen, or 123 every 334. The last is not very practical. Some Greek cities used three every eight. That already is a lot more accurate than two every five -- if Balsam (or the Mahâbhârata) was talking about lunar months.
Three months in every eight years results in an error of a month in 149 years, while two in every five results in an error of a month in only 32 years. That is better than one in every three, which is off a month in 29 years, but otherwise looks pretty miserable. Four months every eleven years results in an error of a month in 216 years; and seven every nineteen results in an error of a month in 6494 years. For all practical purposes, of course, 6494 years is eternity. That does not mean that the Babylonian (or Jewish) calendar is just fine for that long. Those calendars can be adjusted before an error of an entire month builds up. But Indian calendars using the 2/5 rule are going to be wildly inaccurate before the passage of much time at all. Of course, one problem with sources like Balsam and Sen is that they don't seem very aware of differences between lunar and solar calendars. Both were used in India. But while the Mahâbhârata rule (at least as stated by Sen) looks tailored for a solar calendar, a luni-solar calendar, with lunar months and intercalations, looks to be older and more indigenous.
The names of the lunar months are given with dates of the Gregorian year. This represents the adaptation of the calendar to the tropical year as formulated in an official Indian Government calendar reform in 1957. With this calendar now pegged to the Gregorian, any mysteries and peculiarities about its use disappear. Two dates are given in March because the year begins on March 21 in Gregorian leap years, March 22 otherwise. These months now correspond to the signs of the Zodiac (with the Vernal Equinox in Aries), unlike the traditional calendar, which used the actual constellations of the Zodiac (where the Vernal Equinox is now in Pisces, moving into Aquarius).
Part of the calendar reform was the official adoption of the Saka Era, Shakakâla, 
 . The Astronomical Almanac always gives the Era of the Indian calendar thus, with its New Year as specified in the table [U.S. Government Printing Office, Washington, and Her Majesty's Stationery Office, London]. The Almanac for 2001, for instance, cites the New Year on March 22 and the year as 1923 [p.B2]. Amartya Sen mentions that the use of the Saka Era is first attested in an inscription from 543 AD (Saka year 465), at the very end of the Gupta period [p.326].
. The Astronomical Almanac always gives the Era of the Indian calendar thus, with its New Year as specified in the table [U.S. Government Printing Office, Washington, and Her Majesty's Stationery Office, London]. The Almanac for 2001, for instance, cites the New Year on March 22 and the year as 1923 [p.B2]. Amartya Sen mentions that the use of the Saka Era is first attested in an inscription from 543 AD (Saka year 465), at the very end of the Gupta period [p.326].
The Los Angeles Times of November 17, 2001, says that the Indian New Year occurred the previous day, November 16, and that it began the year 2058 -- signifying an Era benchmarked at 57 BC. This would be the Vikrama Era, a historical alternative to the Saka and other Indian eras, with a New Year reckoned from the lunar month of  ; but the Times article contains no discussion of what the Era is or about possible alternates. The Times article referenced the New Year celebration at the cited Hindu temple in Los Angeles, thus raised more questions than it answered. Probably the temple was using one of the traditional, regional Indian calendars, of which there are many, covered by Indian publications but not even by the The Oxford Companion to the Year.
; but the Times article contains no discussion of what the Era is or about possible alternates. The Times article referenced the New Year celebration at the cited Hindu temple in Los Angeles, thus raised more questions than it answered. Probably the temple was using one of the traditional, regional Indian calendars, of which there are many, covered by Indian publications but not even by the The Oxford Companion to the Year.
| Solar/Zodiacal Month or Rasi | |
|---|---|
 |  Aries Aries |
 |  Taurus Taurus |
 |  Gemini Gemini |
 |  Cancer Cancer |
 |  Leo Leo |
 |  Virgo Virgo |
 |  Libra Libra |
 |  Scorpio Scorpio |
 |  Sagittarius Sagittarius |
 |  Capricorn Capricorn |
 |  Aquarius Aquarius |
 |  Pisces Pisces |
This is the calendar apparently developed by Gupta era astronomers mentioned by Amartya Sen, like the mathematician and astronomer Âryabhaṭa (in 499 AD). Sen is aware that it drifted against the tropical year but does not seem to realize that this is an artifact of its direct use of the stars, which implies a sidereal rather than tropical standard. Also, since it is based on the direct use of the stars, there is no general calendar rule for it, and it depended,
| Days of the Week | |
|---|---|
 |  Sunday Sunday |
 |  Monday Monday |
 |  Tuesday Tuesday |
 |  Wednesday Wednesday |
 |  Thursday Thursday |
 |  Friday Friday |
 |  Saturday Saturday |
The seven days of the week were also imported, named, as in Latin, after the planets, in the same sequence used there.
Amartya Sen's examination of calendars includes the Vikrama and Saka Eras but also the Kollam and Bengali San Eras, which are benchmarked at 824 AD and 593 AD respectively -- i.e. subtract those numbers from the AD year for the appropriate calendar year. The oldest Eras he gives are for the Buddha Nirvâṇa calendar, benchmarked at 544 BC (add to the AD year), and the Kaliyurga calendar, benchmarked at 4001 BC. Although Sen is aware that the Kaliyurga Era does not date any historical event, he does not explain all the different versions of the calendar cycles, and the system into which the Kaliyurga, as a cyclical period, is embedded. That is all treated here in a footnote to the page on the devotionalistic Gods of Hinduism. Also, we have the anomaly that the beginning of the Kaliyurga period, cited by Sen from Whitaker's Almanack, was a thousand years later as attested by the Arab historian al-Bîrûnî (973-1048). This is perplexing. Sen is aware of the problem, citing [p.323] Âryabhaṭa that the Kaliyurga benchmark was more like 3101 BC (probably al-Bîrûnî's own source). Sen says that the older benchmark is "quite widely used" but does not or cannot account for the origin of the convention. Sen's Buddhist Era (from Whitaker's Almanack again) also seems to differ by a year from the Era of Buddhism used in Thailand, benchmarked at 543 BC. As it happens, The Oxford Companion to the Year also has the Era of Buddhism beginning in 543, so perhaps some small confusion accounts for the difference -- the Oxford Companion itself says that the Era is "elapsed" years since 544, which may leave readers not realizing that 544 would be year 0, with year 1 delayed until 543.

Cycles of Time in Hinduism and Buddhism
Philosophy of Religion, Hinduism
Philosophy of Religion, Calendars
Philosophy of History, Calendars
While modern Irân has become a fiercely Islâmic country, it retains some elements to remind us of its previous religion, Zoroastrianism. Thus, a very common male given name is Of great interest is the continuation in modern Irân of the ancient Zoroastrian calendar. While the religious Islâmic calendar is of course used in Irân, the ancient solar calendar also continues to be used as a civil calendar.

Iranian Calendars

 , Mehrdâd, which actually means "given by Mithra," Mithra being a god even of pre-Zoroastrian Irân (
, Mehrdâd, which actually means "given by Mithra," Mithra being a god even of pre-Zoroastrian Irân ( , Mitra in the Vedas). There are even versions of the same name in Greek and Latin: Μιθριδάτης, Mithridates.
, Mitra in the Vedas). There are even versions of the same name in Greek and Latin: Μιθριδάτης, Mithridates.
| Avestan | Middle Persian, Pahlavi | Modern Persian | days |
|---|---|---|---|
| Fravashinâm | Fravardîno |  Farvardin Farvardin | 31 |
| Ashahê Vahistahê | Ardavahist |   Ordi Behesht Ordi Behesht | 31 |
| Haurvatâto | Horvadad |  Khordâd Khordâd | 31 |
| Tistryêhe | Tîr | 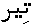 Tir Tir | 31 |
| Amerotâto | Amerôdad |  Mordâd Mordâd | 31 |
| Khshathrahê Vairyêhe | Shatvaîrô |  Shahrivar Shahrivar | 31 |
| Mitrahê | Mitrô |  Mehr Mehr | 30 |
| Apâm | Âvân |  Âbân Âbân | 30 |
| Âthrô | Âtarô |  Âzar Âzar | 30 |
| Dathushô | Dînô |  Dei Dei | 30 |
| Vanheus Mananho | Vohumân |  Bahman Bahman | 30 |
| Spentayâo Armatôis | Spendarmad |  Esfand Esfand | 29/30 |
 , Pahlavi ("Parthian"), the language of the Sassanid Empire, and in Modern Persian (
, Pahlavi ("Parthian"), the language of the Sassanid Empire, and in Modern Persian ( , Fârsi), as used today. Many of the Avestan names are identifiable as relating to what have been called the Zoroastrian Archangels, and to some familar, pre-Zoroastrian gods (Mithra again). The spelling for Modern Persian indicates the Persian vowel quality, not, as is common, the vowels as they would be read in Arabic.
, Fârsi), as used today. Many of the Avestan names are identifiable as relating to what have been called the Zoroastrian Archangels, and to some familar, pre-Zoroastrian gods (Mithra again). The spelling for Modern Persian indicates the Persian vowel quality, not, as is common, the vowels as they would be read in Arabic.
The Irânian year begins with the Vernal Equinox, March 20 or 21. This Persian New Year, 
 , Nouruz (literally, "New Day"), is often celebrated by Irânian expatriates as their distinctively national holiday. The assignment of the lengths of the months reflects the fact that spring and summer in the northern hemisphere are longer than fall and winter. The Persian months are thus actually zodiacal months, comparable to the Chinese Solar Terms. The twelfth month is 29 days in common years, 30 days in leap years. My source (A.K.S. Lambton, Persian Grammar, Cambridge University Press, 1967, p.255-256) does not specify in what year the extra day is added or whether the intercalation scheme is merely Julian or if the Gregorian or some other correction is now applied.
, Nouruz (literally, "New Day"), is often celebrated by Irânian expatriates as their distinctively national holiday. The assignment of the lengths of the months reflects the fact that spring and summer in the northern hemisphere are longer than fall and winter. The Persian months are thus actually zodiacal months, comparable to the Chinese Solar Terms. The twelfth month is 29 days in common years, 30 days in leap years. My source (A.K.S. Lambton, Persian Grammar, Cambridge University Press, 1967, p.255-256) does not specify in what year the extra day is added or whether the intercalation scheme is merely Julian or if the Gregorian or some other correction is now applied.
The Era used with this solar calendar is still the Islâmic  , Hijrah, Era, but it is counted in full solar (365 day) rather than in the short lunar (354 day) years of the Islâmic calendar proper. This means that the Persian year beginning on March 21 can be determined just by subtracting 621 from the AD Era year. Thus, the Persian New Year in 1999 began the solar Hegira year 1378. As discussed elsewhere, this solar Hegira era is equivalent to the year of the Era of Nabonassar (747 BC) minus 1368. Since, astronomically, the Babylonian year also began at the Vernal Equinox, the Babylonian year of the Era of Nabonassar can always be obtained just by adding 1368 to the Irânian solar Hegira year -- 1999 is 1378 + 1368 = 2746 Anno Nabonassari.
, Hijrah, Era, but it is counted in full solar (365 day) rather than in the short lunar (354 day) years of the Islâmic calendar proper. This means that the Persian year beginning on March 21 can be determined just by subtracting 621 from the AD Era year. Thus, the Persian New Year in 1999 began the solar Hegira year 1378. As discussed elsewhere, this solar Hegira era is equivalent to the year of the Era of Nabonassar (747 BC) minus 1368. Since, astronomically, the Babylonian year also began at the Vernal Equinox, the Babylonian year of the Era of Nabonassar can always be obtained just by adding 1368 to the Irânian solar Hegira year -- 1999 is 1378 + 1368 = 2746 Anno Nabonassari.
| Month | Length | Date | |
|---|---|---|---|
| 1 |  Bahâʾ Bahâʾ | 19 | March 21 |
| 2 |  Jalâl Jalâl | 19 | April 9 |
| 3 |  Jamâl Jamâl | 19 | April 28 |
| 4 |  ʿAẓamat | 19 | May 17 |
| 5 |  Nûr Nûr | 19 | June 5 |
| 6 |  Raḥmat | 19 | June 24 |
| 7 |  Kalimât | 19 | July 13 |
| 8 |  Kamâl | 19 | August 1 |
| 9 |  ʾAsmâʾ | 19 | August 20 |
| 10 |  ʿIzzat ʿIzzat | 19 | September 8 |
| 11 |  Mashîyyat | 19 | September 27 |
| 12 |  ʿIlm ʿIlm | 19 | October 16 |
| 13 |  Qudrat Qudrat | 19 | November 4 |
| 14 |  Qawl Qawl | 19 | November 23 |
| 15 |  Masâʾil | 19 | December 12 |
| 16 |  Sharaf | 19 | December 31 |
| 17 |  Sulṭân | 19 | January 19 |
| 18 |  Mulk Mulk | 19 | February 7 |
  ʾAyyâmu-l-Hâʾ [intercalation] | 4/5 | February 26 | |
| 19 |  ʿAlâʾ ʿAlâʾ | 19 | March 2 |

 , (the Splendor of God), was exiled from Irân and imprisoned by Turkey in Haifa. There he was buried after his death in 1892, and there the international headquarters of the Faith is located, while the city itself has passed from Turkey to British Palestine and now to the State of Israel.
, (the Splendor of God), was exiled from Irân and imprisoned by Turkey in Haifa. There he was buried after his death in 1892, and there the international headquarters of the Faith is located, while the city itself has passed from Turkey to British Palestine and now to the State of Israel.
The Bahâʾi calendar has the unique structure of being divided into 19 months of 19 days each. This only falls 4 days short of a 365 day year, which is filled in with intercalary days. The names of the months are given with their Arabic vowel quality, since they are all Arabic words. The intercalary period has been located so that, if the dates given in the table are observed, an intercalation by the Gregorian calendar on February 29 will automatically produce a Bahâʾi intercalary period of 5 rather than 4 days.
The Bahâʾi Faith, although owing much to Islâm, and especially to Irânian Islâm, sees itself as a separate religion that is the successor to Islâm, as Christianity saw itself as the successor to Judaism -- without, however, rejecting the legitimacy of the earlier religions. Unfortunately, to the Irânian authorities, especially after the advent of the "Revolutionary" Irânian theocracy, this meant that Bahâ'is were actually apostates from Islâm, a crime punishable by death under Islâmic Law. Thus, after 1979, all the Bahâʾi holy places in Irân were systematically destroyed and an intense persecution of members of the Faith begun. Many, consequently, fled the country as quickly as possible.
The Faith had long seen itself, however, as an international religion, and communities had long been established all over the world. A local Bahâʾi community had been founded in Hawai'i, for instance, while it was still an independent country. Persecution in Irân, therefore, is liable to be of little significance for the growth of the religion. Indeed, air travelers approaching O'Hare International Airport, in Chicago, are often curious what the unusual large building is on the shore of Lake Michigan. It is the Bahâʾi Temple, in Wilmette, Illinois, which has existed since the early days of the century.
Irânian calendars thus present us with intriguing combinations of pre-Islâmic, Islâmic, and post-Islâmic features, even as Irânian nationalism struggles violently with its own identity and its own religious heritage.  In the Persian national epic, the
In the Persian national epic, the 
 , Shâh Nâmah of the poet Firdawsî (c.940-c.1020), one of the first books written in New (i.e. Islâmic) Persian, there is a striking image from a dream: Four men pulling hard on the corners of a square white cloth, but the cloth does not tear. The men are interpreted to be Moses, Jesus, Muḥammad, and Zoroaster -- Zaraθuštra in Avestan, with the /z/ as in English, the θ in as English "thin," and š as English "sh"; Zartošt, Zardošt, Zardohašt, Zarâdošt (
, Shâh Nâmah of the poet Firdawsî (c.940-c.1020), one of the first books written in New (i.e. Islâmic) Persian, there is a striking image from a dream: Four men pulling hard on the corners of a square white cloth, but the cloth does not tear. The men are interpreted to be Moses, Jesus, Muḥammad, and Zoroaster -- Zaraθuštra in Avestan, with the /z/ as in English, the θ in as English "thin," and š as English "sh"; Zartošt, Zardošt, Zardohašt, Zarâdošt ( ), etc. in Modern Persian -- and the cloth the Religion of God. The inclusion of Zoroaster with the other principal Founders of Monotheism is the distinctively Irânian touch, as Irân itself could be the cloth, pulled fiercely by both internal and external religious influences -- though ironically the word for "religion" in Arabic itself,
), etc. in Modern Persian -- and the cloth the Religion of God. The inclusion of Zoroaster with the other principal Founders of Monotheism is the distinctively Irânian touch, as Irân itself could be the cloth, pulled fiercely by both internal and external religious influences -- though ironically the word for "religion" in Arabic itself,  , dîn, appears to be borrowed from Middle Persian (dên). This image actually has an un-Islamic implication, since it implies equality between the four prophets, while Islam sees Muḥammad as superior to any others, the "Seal of the Prophets," and the only one to deliver a complete and uncorrupted revelation. And Zoroaster has no status or acknowledgement in the Qurʾân or Traditions of the Prophet. Indeed, most Zoroastrians have fled Irân for India and elsewhere, while the theocratic regime in Irân has prohibited the distinctive "Towers of Silence" used for Zoroastrian sky burial.
, dîn, appears to be borrowed from Middle Persian (dên). This image actually has an un-Islamic implication, since it implies equality between the four prophets, while Islam sees Muḥammad as superior to any others, the "Seal of the Prophets," and the only one to deliver a complete and uncorrupted revelation. And Zoroaster has no status or acknowledgement in the Qurʾân or Traditions of the Prophet. Indeed, most Zoroastrians have fled Irân for India and elsewhere, while the theocratic regime in Irân has prohibited the distinctive "Towers of Silence" used for Zoroastrian sky burial.

Philosophy of History, Calendars
Zoroastrianism under the Achaemenids
The Zoroastrian Immortals and Elements
Zoroastrianism under the Sassanids
Philosophy of Religion, Calendars