7 Error Functions, Dawson’s and Fresnel Integrals Properties 7.18 Repeated Integrals of the Complementary Error Function 7.20 Mathematical Applications

§7.19 Voigt Functions

ⓘ

Permalink:: http://dlmf.nist.gov/7.19
See also:: Annotations for Ch.7

§7.19(i) Definitions

ⓘ

Keywords:: Voigt functions, definition, error functions, line broadening function, relation to line broadening function, relations to other functions
Notes:: (7.19.3) follows from (7.2.3) and (7.7.2).
Permalink:: http://dlmf.nist.gov/7.19.i
See also:: Annotations for §7.19 and Ch.7

For $x\in\mathbb{R}$ and $t>0$ ,

7.19.1		$\mathsf{U}\left(x,t\right)=\frac{1}{\sqrt{4\pi t}}\int_{-\infty}^{\infty}\frac% {e^{-(x-y)^{2}/(4t)}}{1+y^{2}}\,\mathrm{d}y,$
ⓘ Defines: $\mathsf{U}\left(\NVar{x},\NVar{t}\right)$ : Voigt function Symbols: $\pi$ : the ratio of the circumference of a circle to its diameter, $\,\mathrm{d}\NVar{x}$ : differential of $x$ , $\mathrm{e}$ : base of natural logarithm, $\int$ : integral and $x$ : real variable Referenced by: §7.19(iii) Permalink: http://dlmf.nist.gov/7.19.E1 Encodings: TeX, pMML, png See also: Annotations for §7.19(i), §7.19 and Ch.7

7.19.2		$\mathsf{V}\left(x,t\right)=\frac{1}{\sqrt{4\pi t}}\int_{-\infty}^{\infty}\frac% {ye^{-(x-y)^{2}/(4t)}}{1+y^{2}}\,\mathrm{d}y.$
ⓘ Defines: $\mathsf{V}\left(\NVar{x},\NVar{t}\right)$ : Voigt function Symbols: $\pi$ : the ratio of the circumference of a circle to its diameter, $\,\mathrm{d}\NVar{x}$ : differential of $x$ , $\mathrm{e}$ : base of natural logarithm, $\int$ : integral and $x$ : real variable Referenced by: §7.19(iii) Permalink: http://dlmf.nist.gov/7.19.E2 Encodings: TeX, pMML, png See also: Annotations for §7.19(i), §7.19 and Ch.7

7.19.3		$\mathsf{U}\left(x,t\right)+i\mathsf{V}\left(x,t\right)=\sqrt{\frac{\pi}{4t}}e^% {z^{2}}\operatorname{erfc}z,$
$z=(1-ix)/(2\sqrt{t})$ .
ⓘ Symbols: $\mathsf{U}\left(\NVar{x},\NVar{t}\right)$ : Voigt function, $\mathsf{V}\left(\NVar{x},\NVar{t}\right)$ : Voigt function, $\pi$ : the ratio of the circumference of a circle to its diameter, $\operatorname{erfc}\NVar{z}$ : complementary error function, $\mathrm{e}$ : base of natural logarithm, $\mathrm{i}$ : imaginary unit, $x$ : real variable and $z$ : complex variable Referenced by: §7.19(i), §7.22(iv) Permalink: http://dlmf.nist.gov/7.19.E3 Encodings: TeX, pMML, png See also: Annotations for §7.19(i), §7.19 and Ch.7

7.19.4		$H\left(a,u\right)=\frac{a}{\pi}\int_{-\infty}^{\infty}\frac{e^{-t^{2}}\,% \mathrm{d}t}{(u-t)^{2}+a^{2}}=\frac{1}{a\sqrt{\pi}}\mathsf{U}\left(\frac{u}{a}% ,\frac{1}{4a^{2}}\right).$
ⓘ Defines: $H\left(\NVar{a},\NVar{u}\right)$ : line-broadening function Symbols: $\mathsf{U}\left(\NVar{x},\NVar{t}\right)$ : Voigt function, $\pi$ : the ratio of the circumference of a circle to its diameter, $\,\mathrm{d}\NVar{x}$ : differential of $x$ , $\mathrm{e}$ : base of natural logarithm and $\int$ : integral Permalink: http://dlmf.nist.gov/7.19.E4 Encodings: TeX, pMML, png See also: Annotations for §7.19(i), §7.19 and Ch.7

$H\left(a,u\right)$ is sometimes called the line broadening function; see, for example, Finn and Mugglestone (1965).

§7.19(ii) Graphics

ⓘ

Notes:: These graphs were produced at NIST.
Permalink:: http://dlmf.nist.gov/7.19.ii
See also:: Annotations for §7.19 and Ch.7

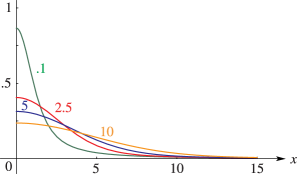
See accompanying text — Figure 7.19.1: Voigt function $\mathsf{U}\left(x,t\right)$ , $t=0.1$ , $2.5$ , $5$ , $10$ . Magnify

§7.19(iii) Properties

ⓘ

Keywords:: Voigt functions, properties
Notes:: (7.19.5) follows from the definitions (7.19.1), (7.19.2), together with (1.17.6) or §2.3(iii). For the first of (7.19.7) use (7.19.1) for the lower bound, and (7.19.10) for the upper bound. For the second of (7.19.7) use (7.19.11). For (7.19.8) and (7.19.9) again use the definitions (7.19.1) and (7.19.2).
Permalink:: http://dlmf.nist.gov/7.19.iii
See also:: Annotations for §7.19 and Ch.7

7.19.5	$\displaystyle\lim_{t\to 0}\mathsf{U}\left(x,t\right)$	$\displaystyle=\frac{1}{1+x^{2}},$
7.19.5	$\displaystyle\lim_{t\to 0}\mathsf{V}\left(x,t\right)$	$\displaystyle=\frac{x}{1+x^{2}}.$
ⓘ Symbols: $\mathsf{U}\left(\NVar{x},\NVar{t}\right)$ : Voigt function, $\mathsf{V}\left(\NVar{x},\NVar{t}\right)$ : Voigt function and $x$ : real variable Referenced by: §7.19(iii) Permalink: http://dlmf.nist.gov/7.19.E5 Encodings: TeX, TeX, pMML, pMML, png, png See also: Annotations for §7.19(iii), §7.19 and Ch.7

7.19.6	$\displaystyle\mathsf{U}\left(-x,t\right)$	$\displaystyle=\mathsf{U}\left(x,t\right),$
7.19.6	$\displaystyle\mathsf{V}\left(-x,t\right)$	$\displaystyle=-\mathsf{V}\left(x,t\right).$
ⓘ Symbols: $\mathsf{U}\left(\NVar{x},\NVar{t}\right)$ : Voigt function, $\mathsf{V}\left(\NVar{x},\NVar{t}\right)$ : Voigt function and $x$ : real variable Permalink: http://dlmf.nist.gov/7.19.E6 Encodings: TeX, TeX, pMML, pMML, png, png See also: Annotations for §7.19(iii), §7.19 and Ch.7

7.19.7	$\displaystyle 0$	$\displaystyle<\mathsf{U}\left(x,t\right)\leq 1,$
7.19.7	$\displaystyle-1$	$\displaystyle\leq\mathsf{V}\left(x,t\right)\leq 1.$
ⓘ Symbols: $\mathsf{U}\left(\NVar{x},\NVar{t}\right)$ : Voigt function, $\mathsf{V}\left(\NVar{x},\NVar{t}\right)$ : Voigt function and $x$ : real variable Referenced by: §7.19(iii) Permalink: http://dlmf.nist.gov/7.19.E7 Encodings: TeX, TeX, pMML, pMML, png, png See also: Annotations for §7.19(iii), §7.19 and Ch.7

7.19.8	$\displaystyle\mathsf{V}\left(x,t\right)$	$\displaystyle=x\mathsf{U}\left(x,t\right)+2t\frac{\partial\mathsf{U}\left(x,t% \right)}{\partial x},$
ⓘ Symbols: $\mathsf{U}\left(\NVar{x},\NVar{t}\right)$ : Voigt function, $\mathsf{V}\left(\NVar{x},\NVar{t}\right)$ : Voigt function, $\frac{\partial\NVar{f}}{\partial\NVar{x}}$ : partial derivative of $f$ with respect to $x$ , $\,\partial\NVar{x}$ : partial differential of $x$ and $x$ : real variable Referenced by: §7.19(iii) Permalink: http://dlmf.nist.gov/7.19.E8 Encodings: TeX, pMML, png See also: Annotations for §7.19(iii), §7.19 and Ch.7
7.19.9	$\displaystyle\mathsf{U}\left(x,t\right)$	$\displaystyle=1-x\mathsf{V}\left(x,t\right)-2t\frac{\partial\mathsf{V}\left(x,% t\right)}{\partial x}.$
ⓘ Symbols: $\mathsf{U}\left(\NVar{x},\NVar{t}\right)$ : Voigt function, $\mathsf{V}\left(\NVar{x},\NVar{t}\right)$ : Voigt function, $\frac{\partial\NVar{f}}{\partial\NVar{x}}$ : partial derivative of $f$ with respect to $x$ , $\,\partial\NVar{x}$ : partial differential of $x$ and $x$ : real variable Referenced by: §7.19(iii) Permalink: http://dlmf.nist.gov/7.19.E9 Encodings: TeX, pMML, png See also: Annotations for §7.19(iii), §7.19 and Ch.7

§7.19(iv) Other Integral Representations

ⓘ

Notes:: See Armstrong (1967).
Permalink:: http://dlmf.nist.gov/7.19.iv
See also:: Annotations for §7.19 and Ch.7

7.19.10		$\mathsf{U}\left(\frac{u}{a},\frac{1}{4a^{2}}\right)=a\int_{0}^{\infty}e^{-at-% \frac{1}{4}t^{2}}\cos\left(ut\right)\,\mathrm{d}t,$
ⓘ Symbols: $\mathsf{U}\left(\NVar{x},\NVar{t}\right)$ : Voigt function, $\cos\NVar{z}$ : cosine function, $\,\mathrm{d}\NVar{x}$ : differential of $x$ , $\mathrm{e}$ : base of natural logarithm and $\int$ : integral Referenced by: §7.19(iii) Permalink: http://dlmf.nist.gov/7.19.E10 Encodings: TeX, pMML, png See also: Annotations for §7.19(iv), §7.19 and Ch.7

7.19.11		$\mathsf{V}\left(\frac{u}{a},\frac{1}{4a^{2}}\right)=a\int_{0}^{\infty}e^{-at-% \frac{1}{4}t^{2}}\sin\left(ut\right)\,\mathrm{d}t.$
ⓘ Symbols: $\mathsf{V}\left(\NVar{x},\NVar{t}\right)$ : Voigt function, $\,\mathrm{d}\NVar{x}$ : differential of $x$ , $\mathrm{e}$ : base of natural logarithm, $\int$ : integral and $\sin\NVar{z}$ : sine function Referenced by: §7.19(iii) Permalink: http://dlmf.nist.gov/7.19.E11 Encodings: TeX, pMML, png See also: Annotations for §7.19(iv), §7.19 and Ch.7

7.18 Repeated Integrals of the Complementary Error Function 7.20 Mathematical Applications

Site Privacy Accessibility Privacy Program Copyrights Vulnerability Disclosure No Fear Act Policy FOIA Environmental Policy Scientific Integrity Information Quality Standards Commerce.gov Science.gov USA.gov

Note: This service is not intended for secure transactions such as banking, social media, email, or purchasing. Use at your own risk. We assume no liability whatsoever for broken pages.

§7.19 Voigt Functions

Contents

§7.19(i) Definitions

§7.19(ii) Graphics

§7.19(iii) Properties

§7.19(iv) Other Integral Representations

Pfad - The Proxy pFad of © 2024 Garber Painting. All rights reserved.