Skip to main content
PMC home page
UKPMC Funders Author Manuscripts logoLink to UKPMC Funders Author Manuscripts
. Author manuscript; available in PMC: 2008 Apr 30.
Published in final edited form as: Psychopharmacology (Berl). 2007 Jul 22;195(1):71–84. doi: 10.1007/s00213-007-0882-0

EFFECTS OF QUINOLINIC ACID-INDUCED LESIONS OF THE NUCLEUS ACCUMBENS CORE ON INTER-TEMPORAL CHOICE

A QUANTITATIVE ANALYSIS

G Bezzina 1, THC Cheung 1, K Asgari 1, CL Hampson 1, S Body 1, CM Bradshaw 1, E Szabadi 1, JFW Deakin 1, IM Anderson 1
PMCID: PMC2092416  EMSID: UKMS1157  PMID: 17659381

Abstract

Rationale

There is evidence that lesions of the nucleus accumbens core (AcbC) promote preference for smaller earlier reinforcers over larger delayed reinforcers in inter-temporal choice paradigms. It is not known whether this reflects an effect of the lesion on the rate of delay discounting, on sensitivity to reinforcer magnitude, or both.

Aim

We examined the effect of AcbC lesions on inter-temporal choice using a quantitative method that allows effects on delay discounting to be distinguished from effects on sensitivity to reinforcer size.

Method

16 rats received bilateral quinolinic acid-induced lesions of the AcbC; 14 received sham lesions. They were trained under a discrete-trials progressive delay schedule to press two levers (A and B) for a sucrose solution. Responses on A delivered 50 μl of the solution after a delay dA; responses on B delivered 100 μl after dB. dB increased across blocks of trials, while dA was manipulated across phases of the experiment. Indifference delay dB(50) (value of dB corresponding to 50% choice of B) was estimated in each phase, and linear indifference functions (dB(50) vs. dA) derived.

Results

dB(50) increased linearly with dA (r2>0.95 in each group). The intercept of the indifference function was lower in the lesioned than the sham-lesioned group; slope did not differ between groups. The lesioned rats had extensive neuronal loss in the AcbC.

Conclusions

The results confirm that lesions of the AcbC promote preference for smaller, earlier reinforcers and suggest that this reflects an effect of the lesion on the rate of delay discounting.

Keywords: quinolinic acid, excitotoxin, lesion, nucleus accumbens, inter-temporal choice, delay of reinforcement, delay discounting, rat

Introduction

In a typical inter-temporal choice situation, an organism is confronted with a choice between two reinforcers that differ in size and delay. For example, response A may deliver a smaller reinforcer after a shorter delay, and response B a larger reinforcer after a longer delay. In such situations, choice is jointly determined by the sizes and delays of both reinforcers (Rachlin 1974; Ainslie 1975; Herrnstein 1981; Green et al. 1981; Mazur 1987; Logue 1988; Ho et al. 1999).

The neuroanatomical substrate of inter-temporal choice has been the subject of much recent interest (for reviews, see Cardinal et al. ​2003​, ​2004​; Cardinal 2006). Among the structures that have been implicated in inter-temporal choice is the nucleus accumbens core (AcbC) (Cardinal et al. ​2001, ​2003​; Pothuizen et al. 2005​; Acheson et al. 2006). Cardinal et al. (2001) found that selective lesions of the AcbC in the rat induced a decrease in preference for a larger/delayed reinforcer over a smaller/immediate reinforcer. Cardinal et al. (2001) suggested that the AcbC may play an important role in the regulation of delay discounting (the hypothetical decline of reinforcer value as a function of delay: see Rachlin 2006). However, the experimental design used in Cardinal et al.’s (2001) experiment permits an alternative explanation, namely that the lesion may have altered the rats’ sensitivity to the different reinforcer magnitudes associated with the two response alternatives (see Cardinal et al. 2003). Subsequent experiments have yielded mixed results, some favouring a role of the AcbC in delay discounting (Pothuizen et al. 2005) and others not (Acheson et al. 2006).

The present experiment re-examined the effect of destruction of the AcbC on inter-temporal choice using a quantitative approach which allows effects on delay discounting to be dissociated from effects on sensitivity to reinforcer magnitude. The approach is based on the multiplicative hyperbolic model of inter-temporal choice proposed by Ho et al. (1999). According to this model, the value of a reinforcer (V) is established by the multiplicative combination of two or more hyperbolic discounting functions that influence the effects of salient features of the reinforcer, including its size (q) and delay (d):

V=11+Q∕q.11+K.d [1]

Q and K are discounting parameters that define an organism’s sensitivity to reinforcer size and the rate of delay discounting respectively (Ho et al. 1999). In an inter-temporal choice situation in which the organism chooses between reinforcer A, of size qA and delay dA, and reinforcer B, of size qB and delay dB, preference for A is assumed to imply that VA>VB, and vice versa, while indifference (i.e. equal choice of A and B) implies that VA=VB. Focusing on indifference points allows the hypothetical entity ‘value’ to be dropped from the equation. Moreover, simple null equations may be derived which allow changes in the delay- and size-discounting parameters to be distinguished. For example, expansion of the indifference relation, VA=VB using equation 1, and solving for dB(50) (the value of dB at the point of indifference) yields the following linear relation between dB(50) and dA:

dB(50)=1K.[11+Q∕qB−11+Q∕qA11+Q∕qA]+dA.[1+Q∕qA1+Q∕qB] [2]

The slope of the function, which defines the ratio of the instantaneous values of the two reinforcers, is influenced by Q, but not by K. The intercept, on the other hand, is jointly determined by Q and K. It follows, therefore, that an intervention which selectively alters the delay discounting parameter K, should alter the intercept of the indifference function (see Ho et al. 1999).

The present experiment employed this approach to examine the effect of lesions of the AcbC on inter-temporal choice. Bilateral lesions of the AcbC were induced by injection of the excitotoxic N-methyl-D-aspartate receptor agonist quinolinic acid (Schwarcz et al. 1983). Lesioned and sham-lesioned rats were trained to steady state in a discrete-trials progressive delay schedule similar to that described by Evenden and Ryan (1996). In every session, the delay to the larger reinforcer (dB) was increased progressively across blocks of trials, allowing an estimation dB(50). The delay to the smaller reinforcer (dA) was changed across successive phases of the experiment, allowing the linear function between dB(50) and dA (equation 2) to be derived.

Method

The experiment was carried out in accordance with UK Home Office regulations governing experiments on living animals.

Subjects

Thirty experimentally naïve female Wistar rats obtained from Charles River Laboratories UK, aged approximately 15 weeks and weighing between 250-300g at the start of the experiment, were individually housed under a constant cycle of 12 hours of light and 12 hours of darkness (lights on 0600-1800 hours) and controlled temperature (21±2°C) and humidity (50±10%). The rats were kept at 80% of their initial free-feeding body weight throughout the experiment by providing a limited amount of standard rodent diet after each experimental session. Tap water was available ad libitum in the home cages.

Apparatus

The rats were trained in standard operant conditioning chambers (CeNeS Ltd, Cambridge, UK) of internal dimensions 25 × 25 × 22 cm. One wall of the chamber contained a recess covered by a hinged clear Perspex flap, into which a peristaltic pump could deliver a 0.6 M sucrose solution. Two apertures situated 5 cm above and 2.5 cm to either side of the recess allowed the insertion of motorised retractable levers (CeNeS Ltd, Cambridge, UK) into the chamber. The levers could be depressed by a force of approximately 0.2 N. A 2.8-W lamp was mounted 2.5 cm above each lever; a third lamp was mounted 10 cm above the central recess. Six red light-emitting diodes were mounted in a row, 4 cm apart, 5 cm above the levers. The operant chamber was enclosed in a sound-attenuating chest with additional masking noise generated by a rotary fan. An Acorn® microcomputer programmed in Arachnid BASIC (CeNeS Ltd, Cambridge, UK), located in an adjoining room, controlled the schedules and recorded the behavioural data.

Surgery

The rats received either bilateral lesions of the AcbC (n = 16) or sham lesions (n=14). Each rat was anaesthetized with halothane (4% in oxygen) and placed in a stereotaxic frame (David Kopf Instruments, Tujunga, CA) using atraumatic ear bars, with the incisor bar set 3.3 mm below the inter-aural line. Anaesthesia was maintained with 2.5% halothane in oxygen during surgery. A small hole was drilled in the skull over each hemisphere to allow insertion of a 0.3-mm diameter cannula connected by a polythene tube to a 10-μl Hamilton syringe. The target stereotaxic co-ordinates (mm, measured from bregma) for the AcbC were: AP +1.2, L ±1.8, V -7.1 (Paxinos and Watson 1998). In the case of the lesioned group, the cannula tip was lowered to the target position in one hemisphere, and 0.5 μl of quinolinic acid (0.1 M in phosphate buffered 0.9% saline solution, pH 7.0) was injected at a rate of 0.1 μl per 20 s. The cannula was left in position for 3 minutes after the completion of the injection, and was then withdrawn. The procedure was then repeated in the other hemisphere. The rats in the sham-lesioned group underwent the same procedure, except that the vehicle alone was injected.

Behavioural training

Fourteen days after surgery, the rats were put on a food-deprivation regimen and their weights gradually reduced to 80% of their free-feeding body weights. They were then trained to press two levers (A and B) for sucrose reinforcement, and were exposed to a discrete-trial continuous reinforcement schedule for two sessions. Subsequently, they underwent daily training sessions under the discrete-trials delayed reinforcement schedule. Each experimental session consisted of six blocks of six trials, except in phases 4 and 5 when sessions consisted of five blocks. The trials were 90 s in duration, with the exception of phase 5, in which the duration was increased to 120 s in order to accommodate the long delay to reinforcement (see below). The six blocks were signalled by illumination of the six light-emitting diodes: in block 1 the first (left-most) diode was illuminated, in block 2 the first and second diodes were illuminated, and so on. The first two trials of each block were forced-choice trials in which each lever was presented alone in random sequence. The other four trials were free-choice trials in which both levers were presented. The beginning of each trial was signalled by illumination of the central light above the reinforcer recess. After 2.5 s the lever or levers (depending on the type of trial) were inserted into the chamber. At the occurrence of a lever-press, the lever(s) were withdrawn, the central light was extinguished, and the light located above the lever that had been depressed was illuminated. This light remained illuminated until the delivery of the reinforcer, and was then extinguished. The chamber remained in darkness until the start of the following trial. If no lever-press occurred within 5 s of the lever(s) being inserted, the lever(s) were retracted and the central light extinguished. (This seldom happened except during the first few training sessions.) A response on lever A initiated a fixed delay dA, following which 50 μl of the 0.6 M sucrose solution was delivered. A response on lever B initiated a variable delay dB (see below), after which 100 μl of the same sucrose solution was delivered. The positions of levers A and B (left vs. right) were counterbalanced across subjects.

The order of conditions is shown in Table 1. The main experiment consisted of six phases, in which the value of dA was set at 1, 2, 4, 8, 12 and 0.5 s, respectively. In each phase, the value of dA was held constant. In each session the value of dB was set equal to dA in the first block of trials. In subsequent blocks dB was increased in increments of 75%. In phases 4 and 5, when dA was 8 s and 12 s, respectively, computing five increments of 75% would have generated a value of dB that was longer than the duration of a trial in the sixth block of trials; therefore the number of blocks was limited to five in these phases. The first phase continued for 100 sessions, phase 2 for 50 sessions, and the remaining phases for 40 sessions. The number of sessions in each phase was based on our previous experience of obtaining stable preference under this schedule (see Kheramin et al. 2003).

Table 1.

Summary of the experimental conditions in each phasee

Phase of experiment dA (s) dB (s)
 Number of sessions
Block 1 Block 2 Block 3 Block 4 Block 5 Block 6
1 1 1.00 1.75 3.06 5.36 9.38 16.41 100
2 2 2.00 3.05 6.13 10.72 18.76 32.83 50
3 4 4.00 7.00 12.25 21.44 37.52 65.65 40
4 8 8.00 14.00 24.50 42.88 75.03 — 40
5* 12 12.00 21.00 36.75 64.31 112.55 — 40
6 0.5 0.500 0.88 1.53 2.68 4.69 8.21 40
7 0 0 0 0 0 0 0 50
8† 0 0 0 0 0 0 0 50
Open in a new tab
*

The trial length was increased to 120 s in phase 5; in all other phases it was 90 s.

†

The reinforcer magnitudes for A and B were switched in phase 8.

After the completion of the main experiment, there were two further phases, 7 and 8, each consisting of 50 sessions, in which dA and dB were set to 0 s in all six blocks. In phase 8 the the reinforcers associated with levers A and B were switched (i.e., responses on A delivered 100 μl and responses on B 50μl of the sucrose solution). Experimental sessions were carried out 7 days a week, at the same time each day, during the light phase of the daily cycle (between 0800 and 1400 hours).

Histology

At the end of the behavioural experiment, the rats were deeply anaesthetized with sodium pentobarbitone, and perfused transcardially with 0.9% sodium chloride, followed by 10% formol saline. The brains were removed from the skull and fixed in formol saline for 1 week. 40-μm coronal sections were taken through the nucleus accumbens region using a freezing microtome.

Cresyl violet staining

The procedure was the same as that described by Kheramin et al. (2002). Alternate sections were mounted on gelatine-coated slides, and dried in formaldehyde vapour for 24 hours. They were immersed in a succession of descending concentrations of ethanol, stained in 0.25% cresyl violet for 5 minutes at room temperature, dehydrated by successive immersion in ascending concentrations of ethanol, cleared in xylene, and mounted with DPX.

Immunocytochemistry

In the other sections neurone-specific nuclear protein (NeuN) was labelled as described by Jongen-Relo and Feldon (2002). Freshly sliced sections were rinsed in 0.1 M phosphate buffered saline (PBS) and placed in 0.5% H2O2 in 0.1 M PBS for 30 minutes to block endogenous peroxidase activity. The sections were rinsed twice in 0.1 M PBS, and placed for 1 hour in a blocking solution consisting of 10% normal horse serum (Vector Laboratories, Peterborough, UK), 1% bovine serum albumin (BSA, Sigma-Aldrich, Gillingham, UK) and 0.3% Triton X-100 (Sigma-Aldrich) in 0.1 M PBS. The sections were incubated for 48 hours at 4°C with the primary antibody solution consisting of monoclonal mouse anti-NeuN serum (1:5000, Chemicon, Chandlers Ford, UK) in 1% normal horse serum, 1% BSA and 0.3% Triton X-100 in 0.1M PBS. After twice washing in 0.1 M PBS, they were left for 2 hours at room temperature in biotinylated horse antimouse serum (1:1000, Vector Laboratories) in a secondary diluent consisting of 1% BSA and 0.3% Triton X-100 in 0.1 M PBS. The sections were rinsed twice in 0.1 M PBS and placed for 2 hours in avidin-biotin-horseradish peroxidase complex (1:200, ABC-Elite, Vector Laboratories) in 0.1 M PBS. After two further rinses in 0.1 M PBS, they were placed in a chromagen solution consisting of 0.05% diaminobenzidine (Sigma-Aldrich) and 0.01% H2O2 (Sigma-Aldrich) for 5 minutes. The reaction was observed visually and stopped by rinsing the sections in 0.1 M PBS. Finally, the sections were mounted on gelatine-coated slides, air dried and mounted with DPX.

An investigator who was not aware of behavioural results performed the microscopic examination. Drawings of the area of the lesions were superimposed on the appropriate coronal sections in the stereotaxic atlas of Paxinos and Watson (1998).

Data analysis

The immunocytochemistry revealed 3 misplaced lesions in the lesioned group. Of the 13 remaining AcbC-lesioned rats, one rat which displayed persistent exclusive responding on one lever was discarded. This left 12 lesioned and 14 sham-lesioned rats for inclusion in the data analysis. All analyses were carried out on cumulated data from the last 10 sessions of each phase of the experiment. As this encompassed more than two oestrus cycles for each rat, oestrus cycle was not taken into account in the analysis.

Preference functions and linear indifference functions

For each rat, the percentage choice of lever B in the free-choice trials (%B) was computed for each block in each phase of the main experiment (phases 1-6). These data were analysed by 3-way analysis of variance (ANOVA) (group × phase × block) with repeated measures on the second and third factors. Plots of %B vs. dB were derived for each rat, and the indifference delay [dB(50): the value of dB corresponding to %B=50] was estimated by linear interpolation between the two delays which fell on either side of %B=50% (i and j) using the formula: dB(50)=dB(i)+[(dB(j)-dB(i)).(%Bi-50)/(%Bi-%Bj)] (Snedecor and Cochran 1989). The indifference delays dB(50) were subjected to a two-factor ANOVA (group × phase) with repeated measures on the second factor. Plots of dB(50) vs. dA were obtained for each rat, and linear functions were fitted by the method of least squares. The slope and intercept of the linear indifference functions were compared between the two groups using Student’s t-test. As the data showed considerable heteroscedasticity, a further analysis was carried out using linear mixed-effect modelling on logarithmically transformed dB(50) data.

Psychophysical analysis of preference functions

The following two-parameter logisitic equation was fitted to the preference data in each phase of the experiment:

%B=100∕[1+(dB∕dB(50))ε] [3]

This function defines a descending sigmoid curve which is symmetrical in semi-logarithmic co-ordinates; dB(50) and ε are parameters expressing the point of intersection of the curve and the indifference line and the slope of the function, respectively. Equation 3 was fitted by non-linear mixed-effect modelling using the maximum likelihood method (Lindstrom and Bates 1990; Pinheiro and Bates 2004). dB(50) and ε were estimated for each group and the log-likelihood ratio was used to test for an effect of group on the parameter estimates (see Pinheiro and Bates 2004).

A further analysis was carried out to determine whether the effect of the lesion on the %B data could be accounted for by a downward displacement of the preference function without any change in the rate of delay discounting (see Discussion for details). The following modified logistic equations were fitted:

%B=100∕[1+(dB∕dB(50))ε]−b [4]
%B=max∕[1+(dB∕dB(max∕2))ε] [5]

The subtractive parameter, b, in equation 4, and the multiplicative parameter, max, in equation 5, capture the downward displacement of the preference function. (Equation 4 has the drawback of allowing negative values of %B; however it yields a direct estimate of the indifference point, dB(50), whereas the indifference point can only be derived from Equation 5 by substitution: dB(50) = exp[ln({max/50}-1)/ε + ln(dB(max/2))].) Models were compared which allowed b or max to vary between groups while dB(50) was held constant between groups, and vice versa. The likelihood ratio test was used to compare the fits provided by the models (Pinheiro and Bates 2004).

As the results indicated an advantage of equation 3 over equations 4 and 5 (see Results), the parameters of the former equation were used to determine a limen ([dB(25)-dB(75)]/2, where dB(25) and dB(75) are the estimated values of dB corresponding to %B=25 and %B=75, respectively), and Weber fraction (defined as limen/dB(50)) for each rat. The Weber fraction was subjected to ANOVA (group × phase) and linear functions were derived for dB(50) vs. dA, as described above.

Choice in the absence of delays

The mean percentage responding on lever B (%B), averaged across all the blocks in a session, was computed for phases 7 (0-s delays on A and B) and phase 8 (0-s delays on A and B, with reinforcer magnitudes switched). The means were compared between groups using Student’s t-test.

Results

Behavioural Data

Rats in both groups learned to respond on both levers within two or three sessions, seldom failing to respond within 5 s after the levers had been inserted into the chamber. The 3-way analysis of variance (group × phase × block) of the proportional choice of B (%B) revealed significant main effects of group [F(1,24)=13.0, P<0.01], phase [F(5,120)=18.6, P<0.001] and block [F(4,96)=134.9, P<0.001), and significant phase × group [F(5,120=2.6, P<0.05] and phase × block [F(20,480)=11.3, P<0.001] interactions. There were no significant block × group [F(4,96)=1.0, P>0.05] or phase × block × group [F(20,480)=1.2, P>0.2] interactions.

Preference functions and linear indifference functions

Plots of %B vs. dB in each phase are shown in Figure 1 (left-hand panels). In both groups, preference for B declined progressively as a function of dB. The functions were located progressively further towards the right with increasing values of dA. The horizontal lines in the graphs indicate the indifference level (i.e. %B=50); the value of dB at which the preference functions cross this level (i.e dB(50)) was generally lower for the lesioned group than for the sham-lesioned group.

Figure 1.

Figure 1

Open in a new tab

Group mean data from the sham-lesioned group (upper panels) and AcbC-lesioned group (lower panels). Left-hand panels show preference functions (percent responding on lever B, %B, vs. delay to the larger of the two reinforcers after a response on B (dB, s). Each set of points shows data collected from one phase of the experiment, in which the delay to the smaller reinforcer (dA) was set at the value indicated (see inset). The horizontal reference line denotes indifference (%B=50). The intersection between each preference function and the indifference level denotes the indifference delay (dB(50)) for that phase. Right-hand panels show transformations of the preference functions with dB (s) on a logarithmic scale, and fitted logistic psychophysical functions, for each value of dA. Note the leftward displacement and flatter slopes of the functions derived for the AcbC-lesioned group compared to those derived for the sham-lesioned group.

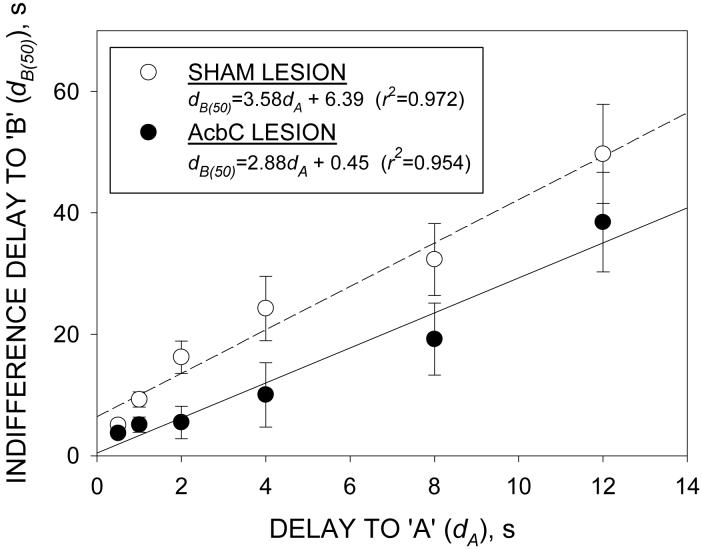
dB(50) was estimated for each rat in each phase. ANOVA of these data (group × phase) yielded significant main effects of both group [F(1,24)=68.8), P<0.001] and phase [F(5,120)=35.0, P<0.001], but no significant group × phase interaction [F(5,120)=1.4, P>0.2]. Plots of dB(50) vs. dA for the group mean data are shown in Figure 2. dB(50) increased linearly with dA in both groups (r2>0.97). The best-fit linear indifference functions were: sham-lesioned group, dB(50)=3.58dA+6.39; lesioned group, dB(50)=2.88dA+0.45 (see inset in Figure 2). Comparisons of the regression functions (Zar 1999) showed no significant difference between the slopes [t(8)=1.5, p>0.2)]. Adopting the common (weighted) slope of 3.23, a t-test on the elevations of the two functions showed a significant difference [t(9)=13.9, p<0.001], corresponding to the lower intercept of the lesioned group’s data.

Figure 2.

Figure 2

Open in a new tab

Linear indifference functions obtained for the sham-lesioned (empty circles) and AcbC-lesioned (filled circles) groups. Ordinate: indifference delay to the larger reinforcer (dB(50), s); abscissa: imposed delay to the smaller reinforcer (dA, s). Points show group mean data; vertical bars indicate SEMs; lines are best-fit linear functions. Equations for the fitted functions: sham-lesioned rats, dB(50)=3.58dA+6.39 (r2>0.97); AcbC-lesioned rats: dB(50)=2.88dA+0.45 (r2>0.95).

Inspection of the data shown in Figure 2 indicated considerable heteroscedasticity, and an analysis using linear mixed-effect modelling was therefore carried out on logarithmically transformed dB(50) data (dA values were also transformed, so as to preserve the theoretical interpretation of the parameters: see Lindstrom and Bates 1990). This analysis showed that the intercept [t(126)=3.8, p<0.001], but not the slope [t(126)=1.03, p>0.3], was significantly affected by the grouping level.

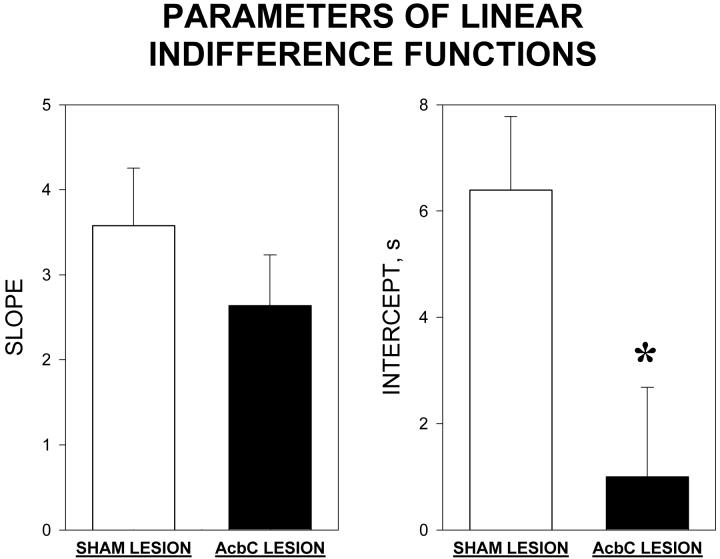
Linear indifference functions were also fitted to the data from the individual rats. The untransformed mean (+SEM) values of the slope and intercept of these function are shown in Figure 3. The slope did not differ significantly between the groups [t(24)=1.0 p>0.1]; however the intercept was significantly higher in the sham-lesioned group than in the AcbC lesioned group [t(24)=2.5 p<0.05].

Figure 3.

Figure 3

Open in a new tab

Parameters of the linear indifference functions from individual rats in the sham-lesioned (open columns) and AcbC-lesioned (filled columns) groups; columns show group mean values, vertical bars indicate SEMs. Left-hand panel: slope of linear function; right-hand panel: intercept of linear function. Significant difference between groups: * P<0.05.

Psychophysical analysis of preference functions

The two-parameter logistic function (equation 3: see Data Analysis) was fitted to the data from each phase of the experiment by non-linear mixed effect modelling using the maximum likelihood approach (see Pinheiro and Bates 2004). In each case this showed a significant influence of subject group on both parameters (dB(50) and ε). Two modified logistic functions were also fitted, which incorporated a third parameter, b (equation 4) or max (equation 5), to capture a downward displacement of the preference functions. Equation 4 produced a significant improvement in fit only in phase 2 (dA = 2 s) [likelihood ratio = 5.58, p<0.05]; in all other phases, no significant improvement of fit was seen over equation 3. Equation 5 produced a significant improvement of fit in phases 1 and 2 (dA = 1 s and 2 s, respectively) [likelihood ratio = 8.94 and 8.10, p<0.01 in each case]; in all other phases, no significant improvement of fit was seen over equation 3. Finally, in the case of each modified logistic equation, two versions incorporating the same number of free parameters were fitted: (i) a grouping factor was incorporated for dB(50), but not for b (equation 4) or max (equation 5); (ii) a grouping factor was incorporated for b (equation 4) or max (equation 5), but not for dB(50). In each phase, the fit provided by the former version of both equation 4 and equation 5 was superior to the latter, as indicated by a smaller sum of squares of the normalized residuals. Accordingly, it was decided that the model that allowed dB(50) to vary between groups was superior to the models that accounted for the between-group differences in preference in terms of ‘downward displacement’ of the preference functions, and therefore the simple two-parameter logistic model (equation 3) was used in the subsequent analyses (see below).

The psychometric curves (equation 3) derived for the group mean data (Figure 1, right-hand panels) accounted for more than 96% of the data variance (r2>0.96) in all cases. The functions derived for the lesioned group were generally flatter and located further to the left than those derived for the sham-lesioned group. Equation 3 could be fitted to 141 of the 156 preference functions obtained for the individual rats in the 6 phases (90.3%). The values of dB(50) derived from the logistic functions were similar to those obtained by linear interpolation (correlation between values obtained by the two methods: sham-lesioned group: r=0.994; AcbC-lesioned group: r=0.985). Neither ε nor the Weber fraction showed a systematic variation across phases [main effect of phase: ε, F(5,120)=1.9, P>0.05; Weber fraction, F(5,120)=1.2, P>0.2]. The group mean values (+SEM) averaged across phases are shown in Figure 4; ε was significantly lower [t(24)=3.6, P<0.01] and the Weber fraction significantly higher [t(24)=3.1, P<0.01] in the lesioned group than in the sham-lesioned group.

Figure 4.

Figure 4

Open in a new tab

Slope parameter (left-hand panel) and Weber fraction (right-hand panel) derived from psychophysical analysis of preference functions (see right-hand panels in Figure 1). See text for derivation of the Weber fraction. Columns show group mean data; vertical bars indicate SEMs. Significant difference between groups: * P<0.01.

Choice in the absence of delays

Figure 5 shows the group mean (+SEM) %B data from the last 10 sessions of phases 7 and 8, when delays to reinforcement were removed (dA = dB = 0). In phase 7, both groups showed a strong preference for lever B, the lever associated with the larger reinforcer. In phase 8, when the reinforcer magnitudes associated with the two levers was reversed, preference switched to lever A. There was no significant difference between the two groups either in phase 7 [t(24)=1.2 p>0.05] or in phase 8 [t(24)=0.3 p>0.2].

Figure 5.

Figure 5

Open in a new tab

Overall percentage preference for the larger reinforcer (%B) when delays to both levers (A and B) were set to 0 s in all blocks of trials. B>A denotes phase 7, where the reinforcer sizes were the same as in the previous 6 phases (B = 100 μl, A = 50 μl); B<A denotes phase 8 where the reinforcer sizes where switched. Columns show group mean data; vertical bars indicate SEMs.

Histology

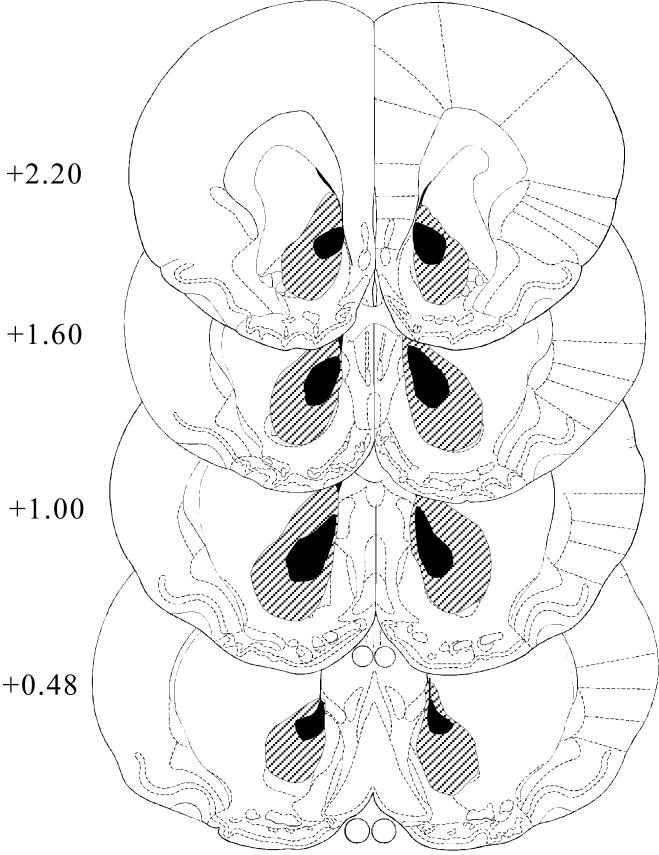
Bilateral lesions of the AcbC were correctly placed in 13 of the 16 rats that received quinolinic acid injections (data from the remaining three rats were discarded; see Methods). Coronal sections showed ventricular dilatation and atrophy in the ventral striatal area. The NeuN labelled sections showed that there was extensive neuronal loss in the area of the AcbC of all lesioned animals, with some neuronal loss in the ventral portion of the caudate-putamen; the shell region of the nucleus accumbens was essentially spared. Figure 6 shows photomicrographs from cresyl violet and NeuN stained sections from a sham-lesioned (upper panels) and a lesioned brain (lower panels). The extent of the lesions is summarized in Figure 7.

Figure 6.

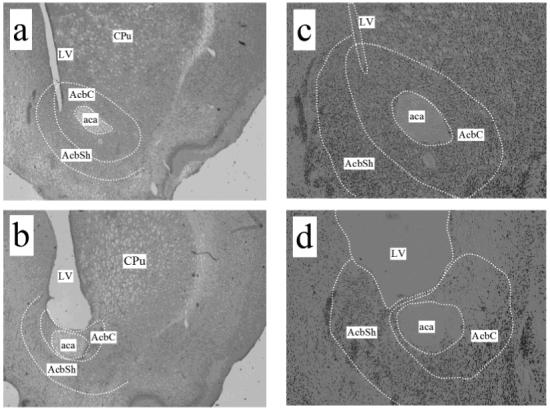
Figure 6

Open in a new tab

Sample photomicrographs showing coronal sections of the brains of a sham-lesioned rat (panels a nand c) and a AcbC-lesioned rat (panels b and d). Left-hand panels: sections stained with cresyl violet; right-hand panels: sections stained for NeuN. LV, lateral ventricle; CPu, caudate-putamen; AcbC, nucleus accumbens core; AcbSh, nucleus accumbens shell; aca, anterior commisure. Note ventricular dilatation, neuronal loss and atrophy of the AcbC in the lesioned brain.

Figure 7.

Figure 7

Open in a new tab

Diagram of approximate area of destruction of the AcbC in the lesioned group. Drawings were made from the microscopic sections, superimposed on the relevant pages of Paxinos and Watson’s (1998) stereotaxic atlas. Locations of the sections in the AP plane (mm anterior to bregma) are indicated on the left. The black area represents the smallest and the shaded area the largest lesion.

Discussion

Injections of quinolinic acid into the AcbC produced lesions of similar extent to those seen in previous experiments in which excitotoxins have been used to lesion this structure (e.g. Bowman and Brown 1998; Cardinal et al. 2001; Pothuizen et al. 2005; Acheson et al. 2006). With the exception of three rats whose data were excluded from the analysis, the lesions were mainly restricted to the AcbC. Some additional damage was inflicted to the ventral portion of the caudate-putamen in some rats; however the mesial shell region of the nucleus accumbens was spared.

The discrete-trials schedule used in this study was an adaptation of the progressive delay schedule developed by Evenden and Ryan (1996). Rats in both the AcbC- and sham-lesioned groups shifted their preference from the larger to the smaller reinforcer as the delay to the larger of the two reinforcers (dB) was progressively increased during a session. This is consistent with previous studies that have used this schedule (Evenden and Ryan ​1996, ​1999; Cardinal et al. ​2000, ​2001; Kheramin et al. ​2002, ​2003, ​2004; Winstanley et al. ​2004, ​2005).

As in previous experiments (Kheramin et al. ​2002​, ​2003​, ​2004), we used a geometric progression (dB=dA×[1.75]n-1) to establish the values of dB in successive blocks within an experimental session, where n denotes the ordinal position of the block. This allowed the range of values of dB to be adapted to the value of dA, which changed across phases 1 to 6 of the experiment. The preference functions (%B vs. dB; see Figure 1) were used to compute the indifference delays to the larger reinforcer (dB(50)) in each phase. This measure formed the basis of the linear indifference functions which, according to the multiplicative hyperbolic model of inter-temporal choice (Ho et al. 1999), allows formal separation of the parameters that represent the rate of delay discounting and sensitivity to reinforcer magnitude (see below).

Models of inter-temporal choice that are based on the computation of hypothetical ‘values’ of reinforcers generally predict extreme preference for the more highly valued of two mutually exclusive reinforcers (see Herrnstein 1981; Ho et al. 1999). This suggests that the ideal preference function should be a step function, %B falling precipitously from near 100% to near 0% around the point at which VA=VB. However, the preference functions in Figure 1 show a gradual reduction of preference as a function of dB, a result that appears to be common to all previous studies employing Evenden and Ryan’s (1996) protocol (Evenden and Ryan ​1996, ​1999; Cardinal et al. ​2000, ​2001; Kheramin et al. ​2002, ​2003, ​2004; Winstanley et al. ​2004, ​2005). This is not simply an artefact of averaging step functions derived from several individuals, because individual rats also show progesssive declines in preference as a function of dB (see below). A possible explanation for this finding is that the gradual decline in preference may represent a discrimination gradient for reinforcer value. Accordingly, we used a standard psychophysical approach to analyse the preference functions. This analysis showed that a two-parameter logistic function adequately described the group mean preference functions (Figure 1, right-hand panels) and approximately 90% of the preference functions obtained from individual rats. The two parameters of this function define its slope (ε) and its locus on the abscissa (dB(50)), which may be used to compute the Weber fraction, the traditional measure of the relative precision of discrimination (see Gibbon ​1977, ​1991; Killeen and Fetterman 1988; Ho et al. 2002; see Killeen 2005, for an example of the derivation of theoretical preference functions based on different theoretical assumptions). It may be noted that the two-parameter logistic function that was fitted to the preference data is a generalized form of a hyperbolic decay function (the logistic function reverts to a simple hyperbola when ε=1). However, notwithstanding its mathematical resemblance to the hypothetical delay discounting function, the preference function is not a simple expression of delay discounting. Indeed, while the delay discouting parameter, K, is believed to be reflected in the indifference point dB(50) (cf. equation 2), it is theoretically unrelated to the slope of the preference function, ε. In other words, a change in K neither predicts, nor is it predicted by, a change in ε. The present results show that the AcbC lesion resulted in a reduction of ε and an increase in the Weber fraction, indicating an impairment of discriminative precision.

One possible interpretation of the effect of the lesion on ε and the Weber fraction is that the AcbC-lesioned rats may have been less able than the sham-lesioned rats to distinguish between the magnitudes of the two reinforcers. Alternatively, the lesion may have impaired the rats’ ability to discriminate the various delays to the larger reinforcer (dB) across successive blocks of trials. The former explanation is rendered implausible by the data from phases 7 and 8, which showed that the AcbC-lesioned rats, like the sham-lesioned rats, showed near-exclusive preference for the larger reinforcer when the delays to reinforcement were removed, and were able to reverse this preference when the assignment of the larger and smaller reinforcers to the two levers was switched. This finding is consistent with previous reports (e.g. Cardinal et al. 2003; Acheson et al. 2006). The alternative proposition, that the AcbC lesion may have impaired the rats’ ability to discriminate within-session changes in delay to reinforcement, is consistent with a recent suggestion by Acheson et al. (2006), and is discussed below.

This study employed a null-equation approach derived from the multiplicative hyperbolic model of inter-temporal choice, which entailed measurement of indifference delays (dB(50)) corresponding to a range of values of dA, which were used to compute linear indifference functions. This method offers the advantage of allowing changes in the delay-discounting (K) and magnitude-sensitivity (Q) parameters in equation 2 to be distinguished. The slope of the linear function defined by equation 2 reflects the physical magnitudes of the two reinforcers (qA and qB) and Q; a change in slope therefore implies a change in Q. The intercept of the function is influenced jointly by Q and K. This means that while an increase in Q causes an increase in both the slope and the intercept, an increase in K simply diminishes the intercept. If both parameters are increased, Q’s effect on the intercept is countered by the change in K, whereas its impact on the slope remains unaltered (Ho et al. 1999).

The slope and intercept of the indifference function seen in the sham-lesioned group are comparable to those seen in previous experiments using the same experimental protocol with rats (Kheramin et al. ​2002, ​2004). The indifference functions of the sham- and AcbC-lesioned groups did not differ significantly in terms of slope; however the function derived for AcbC-lesioned group had a significantly lower intercept than that of the sham-lesioned group. This indicates a selective effect of the lesion on K, the lower intercept corresponding to a higher value of K in the AcbC-lesioned group, in other words, to a higher rate of delay discounting. This result confirms and extends the findings of Cardinal et al. (2001​, 2003), who first reported that AcbC-lesioned rats were more ‘intolerant’ to delay of reinforcement. The use of the indifference-equation approach in the present experiment allows the effect reported by Cardinal et al. to be attributed specifically to an increased rate of delay discounting in AcbC-lesioned rats.

A recent study by Acheson et al. (2006) reported findings that are in apparent contrast with the findings of Cardinal et al. (2001, 2003). Acheson et al. (2006) used an adjusting amount schedule (Richards et al. 1999) in which the delay to a liquid reinforcer of fixed volume was held constant while the volume of an immediate reinforcer was varied in response to the animals’ choices. Acheson et al. (2006) found that while the lesion had no effect on performance when the delay was held constant, lesioned rats showed flatter preference functions when the delay was changed from day to day. Acheson et al. (2006) proposed that the lesion may have disrupted the ability of the rats to adapt to changes in delay, without altering the rate of delay discounting. The present finding of flatter slopes of the psychometric function and larger Weber fractions is consistent with the suggestion that discrimination of within-session changes in dB was less precise in the AcbC-lesioned rats than in the intact rats. However, the lower values of dB(50) in the AcbC-lesioned group suggests that poor discrimination of delays alone cannot account for the lower tolerance of delay shown by the AcbC-lesioned group. It is perhaps surprising that the AcbC-lesioned rats seemed to be less precise in their discrimination of within-session changes in delay, yet, according to the present analysis, they had a higher rate of delay discounting than the intact rats. However, as discussed above, these two postulated effects of the lesion are not incompatible with one another; the rate of delay discounting is believed to reflect a delay-dependent degradation of reinforcer value, whereas the discrimination of delays may reflect non-motivational aspects of interval timing (‘prospective timing’: Killeen et al. 1997). It will be of interest, in future experiments, to examine the effect of AcbC lesions on interval timing behaviour in other schedules that do not entail delay of reinforcement.

As discussed above, the lower values of dB(50) seen in the AcbC-lesioned group is consistent with the notion that the lesion produced an increase in the rate of delay discounting. However, before accepting this interpretation, it is necessary to consider an alternative account. Visual inspection of the preference functions derived from the two groups (Figure 1) indicates that the lesioned group showed weaker preference for the larger reinforcer than the sham-lesioned group, even when the delays to the two reinforcers were equal. This trend, also noted by Cardinal et al. (2001), is reflected in an apparent ‘downward displacement’ of the preference functions derived from the lesioned group compared to those derived from the sham-lesioned group. This raises the possibility that the shorter indifference delays shown by the lesioned group might be explained in terms of a general bias away from the lever associated with longer delays (i.e. lever B), which need not necessarily reflect a higher rate of delay discounting. However, statistical analysis of the preference functions showed that models based on the assumption that the lesion induced a downward displacement of the curve without a concomitant change in delay discounting rate (equations 4 and 5) yielded a less satisfactory account of the data than the model in which the rate of delay discounting was affected by the lesion (equation 3).

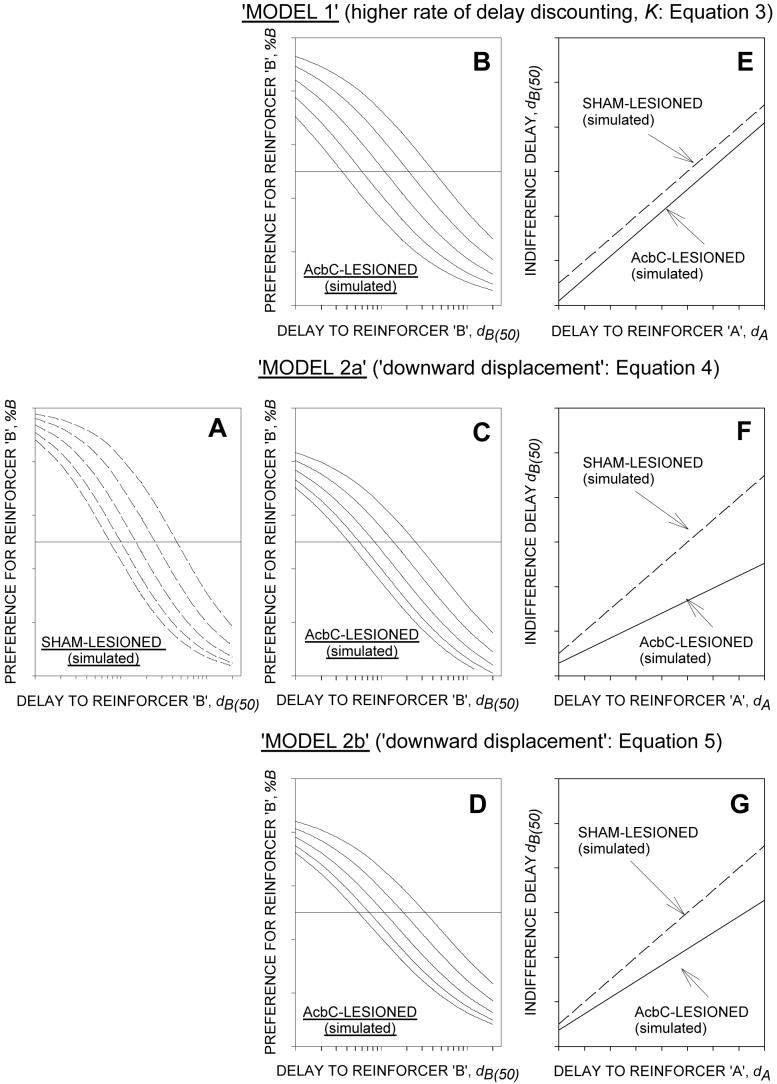
There is also a theoretical reason for doubting whether ‘downward displacement’ per se can account for the shorter indifference delays shown by the AcbC-lesioned group. This is illustrated by the simulated preference and indifference functions in Figure 8. Panel A shows preference functions for a hypothetical sham-lesioned group, and panels B, C and D the corresponding functions for a hypothetical AcbC-lesioned group based on three logistic models. The curves in B (Model 1) are based on the assumption of a higher rate of delay discounting in the lesioned group, whereas the curves in C and D were derived by downward displacement of the preference functions, compared to those of the hypothetical sham-lesioned group (Models 2a and 2b, derived from equations 4 and 5, respectively). Panels E, F and G show the indifference functions corresponding to the preference functions in B, C and D. It can be seen that proportional downward displacement of the preference functions results in a reduction of the slope of the indifference function (F and G), in contrast to the parallel shift of the function that is implied by a higher rate of delay discounting (E). Comparison of these theoretical indifference functions with the empirical functions shown in Figure 2 suggests that the model illustrated in E (faster delay discounting) provides a more veridical depiction of the effect of the lesion than the models illustrated in F and G (downward displacement with no change in discounting rate). Thus, although the possibility that the lesion of the AcbC may have induced some bias away from the lever associated with the longer delays cannot be excluded, the reduction of the intercept of the indifference function with no significant change in slope argues for an increase in the rate of delay discounting in the AcbC-lesioned rats. (The simulated functions in panels B, C and D incorporated a lower value ε than that used to derive the curves shown in Panel A. This was done in order to reflect the empirical finding of a lower value of this parameter in the AcbC-lesioned group than in the sham-lesioned group. Since the same value of ε was used for all the simulated functions shown in panels B, C and D, this has no implications for the differences between the profiles of indifference functions shown in panels E, F and G.)

Figure 8.

Figure 8

Open in a new tab

Theoretical preference and indifference functions, illustrating the implications of three alternative versions of the logistic psychometric preference function for the linear indifference function (see Discussion for details). A: Preference functions (%B vs. dB, in semi-logarithmic coordinates) for a hypothetical sham-lesioned group; each curve corresponds to a different value of dA; the curves were derived from equation 3. B, C and D: Corresponding preference functions for a hypothetical AcbC-lesioned group based on three models. In model 1 (panel B), the curves were derived from equation 3, under the assumption that the location parameter, dB(50), was reduced in the lesioned group due to an increase in the rate of delay discounting. In models 2a and 2b (panels C and D), the curves were derived from equation 4 and 5, under the assumption that the reduction of dB(50) in the lesioned group was brought about by downward displacement of the preference function (in equation 4, downward displacement is generated by a subtractive parameter b [b > 0]; in equation 5, downward displacement is generated by a multiplicative paramater max [max < 100]). Note that the curves in B, C and D are flatter than those in A, reflecting a lower value of ε in hypothetical AcbC-lesioned group, as seen in the present experiment. E, F and G Linear indifference functions corresponding to the preference functions shown in B, C and D. Note that downward displacement of the preference functions results in a reduction of the slope of the indifference function (F and G), in contrast to the parallel shift of the indifference function that is implied by a higher rate of delay discounting (E); the parallel shift is consonant with the empirical finding shown in Figure 2, indicating that model 1 (increased rate of delay discounting) provides a better account of the findings than models 2a and 2b (downward displacement).

It is of interest to compare the present results with previous findings on the effects of orbital prefrontal cortex (OPFC) lesions on inter-temporal choice. Destruction of the OPFC has a dual effect on performance in the progressive delay schedule, steepening the linear indifference function without significantly altering the intercept; at most values of dA the lesion induced an increase in the indifference delay dB(50) (Kheramin et al. ​2002​, ​2003). This effect was interpreted in terms of equation 2 as an increased rate of delay discounting (i.e. an increase of K) coupled with a reduction of instaneous reinforcer values (i.e. an increase in Q) (Kheramin et al. 2005). These findings emphasise the utility of a quantitative approach to inter-temporal choice, since changes in the rate of delay discounting (K) may be associated with either an increase (OPFC lesion: Kheramin et al. ​2002​, ​2003) or a decrease (AcbC lesion: present results) in apparent ‘tolerance to delay’, depending on whether sensitivity to other salient features of reinforcers (e.g. magnitude, manifested in Q) is also affected. Quantitative analysis based on a family of indifference points enabled an effect of both lesions on delay discounting to be revealed (see Mazur 2006).

Comparison of the effects of lesions of the AcbC and OPFC on inter-temporal choice also suggests that considerable caution is needed in the use of terms like ‘impulsiveness’ and ‘self-control’ in this context. Selection of the smaller earlier reinforcer in an inter-temporal choice situation is often deemed to be ‘impulsive’, while selection of the larger delayed alternative is deemed to be ‘self-controlled’. However, apart from their unfortunate anthropomorphic connotations (see Monterosso and Ainslie 1999), these terms can be seriously misleading if they are taken to imply a consistent behavioural tendency linked to the rate of delay discounting. Since inter-temporal choice invariably entails pitting one feature of reinforcers (e.g. delay) against another (e.g. magnitude), a change in the rate of delay discounting is neither a necessary nor a sufficent condition for a shift of choice in the direction of ‘impulsiveness’ or ‘self-control’. This is exemplified by the effects of AcbC and OPFC lesions discussed above, which, depending on the delays and sizes of the reinforcers, may have similar or opposite effects on choice behaviour, although, according to the present analysis, both lesions may have a facilitatory effect on the rate of delay discounting (see Mazur 2006; Bradshaw et al. 2007).

There is growing evidence for the involvement of multiple brain regions in the control of behaviour by delayed reinforcement. In addition to the AcbC (Cardinal et al. ​2001​, ​2003; Pothuizen et al. 2005; present results) and the OPFC (Mobini et al. 2000b; Kheramin et al. ​2002​, ​2003​, ​2004; Winstanley et al. 2004​; Rudebeck et al. 2006), recent evidence implicates the subthalamic nucleus (Winstanley et al. 2005), the basolateral amygdala (Winstanley et al. 2004) and the hippocampus (Cheung and Cardinal 2005). There is also evidence from studies involving acute drug treatment and selective neurotoxins for the involvement of the ascending 5-hydroxytryptaminergic (Wogar et al. 1993​; Evenden and Ryan 1999​; Bizot et al. 1999​; Mobini et al. 2000a) and dopaminergic (Wade et al. 2000​; Cardinal et al. 2000​; Winstanley et al. 2003​; Kheramin et al. 2004) projections to the forebrain. Much further work is needed into how these structures are integrated in the regulation of inter-temporal choice behaviour (see Cardinal 2006).

In conclusion, destruction of the AcbC significantly altered inter-temporal choice behaviour. The results indicated that the lesion promoted the selection of smaller earlier reinforcers in preference to larger delayed reinforcers, in confirmation of earlier reports (Cardinal et al. ​2001​, ​2003). Quantitative analysis of indifference functions indicated that this effect reflected an increase in the rate of delay discounting. In addition, the AcbC lesion may have impaired the ability of the rats to discriminate within-session changes in delay to reinforcement (Acheson et al. 2006).

Acknowledgements

This work was supported by the Wellcome Trust. We are grateful to Ms. V.K. Bak and Mr R.W. Langley for skilled technical help.

References

  1. Acheson A, Farrar AM, Patak M, Hausknecht KA, Kieres AK, Choi S, de Wit H, Richards JB. Nucleus accumbens lesions decrease sensitivity to rapid changes in the delay to reinforcement. Behav Brain Res. 2006;173:217–228. doi: 10.1016/j.bbr.2006.06.024. [DOI] [PMC free article] [PubMed] [Google Scholar]
  2. Ainslie G. Specious reward: a behavioral theory of impulsiveness and impulse control. Psychol Bull. 1975;82:463–496. doi: 10.1037/h0076860. [DOI] [PubMed] [Google Scholar]
  3. Bizot J, Le Bihan C, Puech AJ, Hamon M, Thiebot M. Serotonin and tolerance to delay of reward in rats. Psychopharmacology. 1999;146:400–412. doi: 10.1007/pl00005485. [DOI] [PubMed] [Google Scholar]
  4. Bradshaw CM, Body S, Szabadi E. Decision-making and neuroeconomics: delayed reinforcement, neuroscience. In: Squire L, editor. New encyclopedia of neuroscience, MS#1527. Elsevier; Oxford: 2007. in press. [Google Scholar]
  5. Bowman EM, Brown VJ. Effects of excitotoxic lesions of the rat ventral striatum on the perception of reward cost. Exp Brain Res. 1998;123:439–448. doi: 10.1007/s002210050588. [DOI] [PubMed] [Google Scholar]
  6. Cardinal RN. Neural systems implicated in delayed and probabilistic reinforcement. Neural Networks. 2006;19:1277–1301. doi: 10.1016/j.neunet.2006.03.004. [DOI] [PubMed] [Google Scholar]
  7. Cardinal RN, Pennicott DR, Sugathapala CL, Robbins TW, Everitt BJ. Impulsive choice induced in rats by lesions of the nucleus accumbens core. Science. 2001;292:2499–2501. doi: 10.1126/science.1060818. [DOI] [PubMed] [Google Scholar]
  8. Cardinal RN, Robbins TW, Everitt BJ. The effects of d-amphetamine, chlordiazepoxide, alpha-flupenthixol and behavioural manipulations on choice of signalled and unsignalled delayed reinforcement in rats. Psychopharmacology. 2000;152:362–375. doi: 10.1007/s002130000536. [DOI] [PubMed] [Google Scholar]
  9. Cardinal RN, Robbins TW, Everitt BJ. Choosing delayed rewards: perspectives from learning theory, neurochemistry, and neuroanatomy. In: Heather N, Vuchinich RE, editors. Choice, Behavioral Economics and Addiction. Oxford: Elsevier; 2003. pp. 183–213. [Google Scholar]
  10. Cardinal RN, Winstanley CA, Robbins TW, Everitt BJ. Limbic corticostriatal systems and delayed reinforcement. Ann New York Acad Sci. 2004;1021:33–50. doi: 10.1196/annals.1308.004. [DOI] [PubMed] [Google Scholar]
  11. Cheung THC, Cardinal RN. Hippocampal lesions facilitate instrumental learning with delayed reinforcement but induce impulsice choice in rats. BMC Neurosci. 2005;6:36. doi: 10.1186/1471-2202-6-36. [DOI] [PMC free article] [PubMed] [Google Scholar]
  12. Evenden JL, Ryan CN. The pharmacology of impulsive behaviour in rats: The effects of drugs on response choice with varying delays of reinforcement. Psychopharmacology. 1996;128:161–170. doi: 10.1007/s002130050121. [DOI] [PubMed] [Google Scholar]
  13. Evenden JL, Ryan CN. The pharmacology of impulsive behaviour in rats VI: the effects of ethanol and selective serotonergic drugs on response choice with varying delays of reinforcement. Psychopharmacology. 1999;146:413–421. doi: 10.1007/pl00005486. [DOI] [PubMed] [Google Scholar]
  14. Green L, Fisher EB, Perlow S, Sherman L. Preference Reversal and Self-Control - Choice as a Function of Reward Amount and Delay. Behav Anal Lett. 1981;1:43–51. [Google Scholar]
  15. Gibbon J. Scalar expectancy theory and Weber’s law in animal timing. Psychol Rev. 1977;84:279–325. [Google Scholar]
  16. Gibbon J. Origins of scalar timing. Learn Motiv. 1991;22:3–38. [Google Scholar]
  17. Herrnstein R. Self-control as response strength. In: Bradshaw CM, Szabadi E, Lowe CF, editors. Quantification of steady-state operant behaviour. Amsterdam: Elsevier; 1981. pp. 3–20. [Google Scholar]
  18. Ho MY, Mobini S, Chiang TJ, Bradshaw CM, Szabadi E. Theory and method in the quantitative analysis of “impulsive choice” behaviour: implications for psychopharmacology. Psychopharmacology. 1999;146:362–372. doi: 10.1007/pl00005482. [DOI] [PubMed] [Google Scholar]
  19. Ho M-Y, Velazquez-Martinez DN, Bradshaw CM, Szabadi E. 5-Hydroxytryptamine and interval timing behaviour. Pharmacol Biochem Behav. 2002;71:773–785. doi: 10.1016/s0091-3057(01)00672-4. [DOI] [PubMed] [Google Scholar]
  20. Jongen-Relo AL, Feldon J. Specific neuronal protein: A new tool for histological evaluation of excitotoxic lesions. Physiol Behav. 2002;76:449–456. doi: 10.1016/s0031-9384(02)00732-1. [DOI] [PubMed] [Google Scholar]
  21. Kheramin S, Body S, Ho MY, Velazquez-Martinez DN, Bradshaw CM, Szabadi E, Deakin JFW, Anderson IM. Role of the orbital prefrontal cortex in choice between delayed and uncertain reinforcers: a quantitative analysis. Behav Proc. 2003;64:239–250. doi: 10.1016/s0376-6357(03)00142-6. [DOI] [PubMed] [Google Scholar]
  22. Kheramin S, Body S, Ho MY, Velazquez-Martinez DN, Bradshaw CM, Szabadi E, Deakin JFW, Anderson IM. Effects of orbital prefrontal cortex dopamine depletion on inter-temporal choice: a quantitative analysis. Psychopharmacology. 2004;175:206–214. doi: 10.1007/s00213-004-1813-y. [DOI] [PubMed] [Google Scholar]
  23. Kheramin S, Body S, Miranda Herrera F, Bradshaw CM, Szabadi E, Deakin JFW, Anderson IM. The effect of orbital prefrontal cortex lesions on performance on a progressive ratio schedule: implications for models of inter-temporal choice. Behav Brain Res. 2005;156:145–152. doi: 10.1016/j.bbr.2004.05.017. [DOI] [PubMed] [Google Scholar]
  24. Kheramin S, Body S, Mobini S, Ho MY, Velazquez-Martinez DN, Bradshaw CM, Szabadi E, Deakin JFW, Anderson IM. Effects of quinolinic acid-induced lesions of the orbital prefrontal cortex on inter-temporal choice: a quantitative analysis. Psychopharmacology. 2002;165:9–17. doi: 10.1007/s00213-002-1228-6. [DOI] [PubMed] [Google Scholar]
  25. Killeen PR. Gradus ad Parnassum: Ascending strength gradients or descending memory traces? Behav Brain Sci. 2005;28:432–434. [Google Scholar]
  26. Killeen PR, Fetterman JG. A behavioral theory of timing. Psychol Rev. 1988;95:274–295. doi: 10.1037/0033-295x.95.2.274. [DOI] [PubMed] [Google Scholar]
  27. Killeen PR, Fetterman JG, Bizo LA. Time’s causes. In: Bradshaw CM, Szabadi E, editors. Time and behaviour: psychological and neurobehavioural analyses. Amsterdam: Elsevier; 1997. [Google Scholar]
  28. Lindstrom MJ, Bates DM. Nonlinear mixed-effects models for repeated measures data. Biometrics. 1990;46:673–687. [PubMed] [Google Scholar]
  29. Logue AW. Research on self-control: an integrating framework. Behav Brain Sci. 1988;11:665–678. [Google Scholar]
  30. Mazur JE. An adjusting procedure for studying delayed reinforcement. In: Commons ML, Mazur JE, Nevin JA, Rachlin H, editors. Quantitative Analyses of Behavior: V. The Effect of Delay and of Intervening Events on Reinforcement Value. Hillsdale, New Jersey: Lawrence Erlbaum; 1987. pp. 55–73. [Google Scholar]
  31. Mazur JE. Mathematical models and the experimental analysis of behavior. J Exp Anal Behav. 2006;85:285–291. doi: 10.1901/jeab.2006.65-05. [DOI] [PMC free article] [PubMed] [Google Scholar]
  32. Mobini S, Chiang TJ, Al-Ruwaitea AS, Ho MY, Bradshaw CM, Szabadi E. Effect of central 5-hydroxytryptamine depletion on inter-temporal choice: a quantitative analysis. Psychopharmacology. 2000a;149:313–318. doi: 10.1007/s002130000385. [DOI] [PubMed] [Google Scholar]
  33. Mobini S, Body S, Ho M-Y, Bradshaw CM, Szabadi E. Effects of lesions of the orbitofrontal cortex on sensitivity to delayed and probabilistic reinforcement. Psychopharmacology. 2000b;160:290–298. doi: 10.1007/s00213-001-0983-0. [DOI] [PubMed] [Google Scholar]
  34. Monterosso J, Ainslie G. Beyond discounting: possible experimental models of impulse control. Psychopharmacology. 1999;146:339–347. doi: 10.1007/pl00005480. [DOI] [PubMed] [Google Scholar]
  35. Paxinos G, Watson C. The Rat Brain in Stereotaxic Coordinates. Fourth edn. San Diego: Academic Press; 1998. [Google Scholar]
  36. Pinheiro JC, Bates DM. Mixed-effects models in S and S-plus. New York: Springer; 2004. [Google Scholar]
  37. Pothuizen HHJ, Jongen-Relo AL, Feldon J, Yee BK. Double dissociation of the effects of selective nucleus accumbens core and shell lesions on impulsive-choice behaviour and salience learning in rats. Eur J Neurosci. 2005;22:2605–2616. doi: 10.1111/j.1460-9568.2005.04388.x. [DOI] [PubMed] [Google Scholar]
  38. Rachlin H. Self-control. Behaviorism. 1974;2:94–107. [Google Scholar]
  39. Rachlin H. Notes on discounting. J Exp anal Behav. 2006;85:425–435. doi: 10.1901/jeab.2006.85-05. [DOI] [PMC free article] [PubMed] [Google Scholar]
  40. Richards JB, Sabol KE, de Wit H. Effects of methamphetamine on the adjusting amount procedure, a model of impulsive behavior in rats. Psychopharmacology. 1999;146:432–439. doi: 10.1007/pl00005488. [DOI] [PubMed] [Google Scholar]
  41. Rudebeck PH, Walton ME, Smyth AN, Bannerman DM, Rushworth FS. Separate neural pathways process different decision costs. Nature Neurosci. 2006;9:1161–1168. doi: 10.1038/nn1756. [DOI] [PubMed] [Google Scholar]
  42. Schwarcz R, Whetsell WO, Mangano RM. Quinolinic acid: an endogenous metabolite that produces axon-sparing lesions in rat-brain. Science. 1983;219:316–318. doi: 10.1126/science.6849138. [DOI] [PubMed] [Google Scholar]
  43. Snedecor GW, Cochran WG. Statistical methods. 8th edn. Iowa State University Press; 1989. Iowa State University Press. [Google Scholar]
  44. Wade TR, de Wit H, Richards JB. Effects of dopaminergic drugs on delayed reward as a measure of impulsive behavior in rats. Psychopharmacology. 2000;150:90–101. doi: 10.1007/s002130000402. [DOI] [PubMed] [Google Scholar]
  45. Winstanley CA, Baunez C, Theobald DEH, Robbins TW. Lesions to the subthalamic nucleus decrease impulsive choice but impair autoshaping in rats: the importance of the basal ganglia in Pavlovian conditioning and impulse control. Eur J Neurosci. 2005;21:3107–3116. doi: 10.1111/j.1460-9568.2005.04143.x. [DOI] [PubMed] [Google Scholar]
  46. Winstanley CA, Theobald DEH, Cardinal RN, Robbins TW. Contrasting roles for basolateral amygdala and orbitofrontal cortex in impulsive choice. J Neurosci. 2004;24:4718–4722. doi: 10.1523/JNEUROSCI.5606-03.2004. [DOI] [PMC free article] [PubMed] [Google Scholar]
  47. Winstanley CA, Theobald DEH, Dalley JW, Robbins TW. Global 5-HT depletion attenuates the ability of amphetamine to decrease impulsive choice in rats. Psychopharmacology. 2003;170:320–331. doi: 10.1007/s00213-003-1546-3. [DOI] [PubMed] [Google Scholar]
  48. Wogar MA, Bradshaw CM, Szabadi E. Effects of lesions of the ascending 5-hydroxytryptaminergic pathways on choice between delayed reinforcers. Psychopharmacology. 1993;111:239–243. doi: 10.1007/BF02245530. [DOI] [PubMed] [Google Scholar]
  49. Zar JH. Biostatistical analysis. fourth edition NJ, Prentice-Hall: Upper Saddle River; 1999. [Google Scholar]

RESOURCES

pFad - Phonifier reborn

Pfad - The Proxy pFad of © 2024 Garber Painting. All rights reserved.

Note: This service is not intended for secure transactions such as banking, social media, email, or purchasing. Use at your own risk. We assume no liability whatsoever for broken pages.


Alternative Proxies:

Alternative Proxy

pFad Proxy

pFad v3 Proxy

pFad v4 Proxy